8.2.1 Coordinates, PT3 Focus Practice
Question 1:
In diagram below, Q is the midpoint of the straight line PR.
The value of m is
Solution:
Question 2:
In diagram below, P and Q are points on a Cartesian plane.
If M is the midpoint of PQ, then the coordinates of M are
Solution:
Question 3:
Find the distance between P (–4, 6) and Q (20, –1).
Solution:
Question 4:
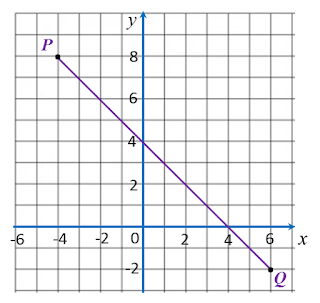
Diagram shows a straight line PQ on a Cartesian plane.
Calculate the length, in unit, of PQ.
Solution:
PS = 15 – 3 = 12 units
SQ = 8 – 3 = 5 units
By Pythagoras’ theorem,
PQ2 = PS2 + SQ2
= 122+ 52
PQ = √169
= 13 units
Question 5:
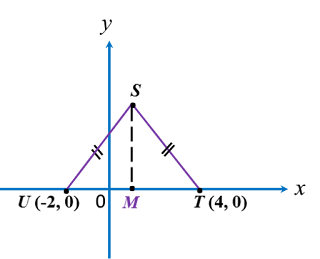
The diagram shows an isosceles triangle STU.
Given that ST = 5 units, the coordinates of point S are
Solution:
For an isosceles triangle STU, M is the midpoint of straight line TU.
Point M = (1, 0)
MT = 4 – 1 = 3 units
By Pythagoras’ theorem,
SM2 = ST2 – MT2
= 52 – 32
= 25 – 9
= 16
SM = √16
= 4
Therefore, point S = (1, 4).