8.2.3 Coordinates, PT3 Focus Practice

Question 11:
Diagram below shows the route of a Negaraku Run.
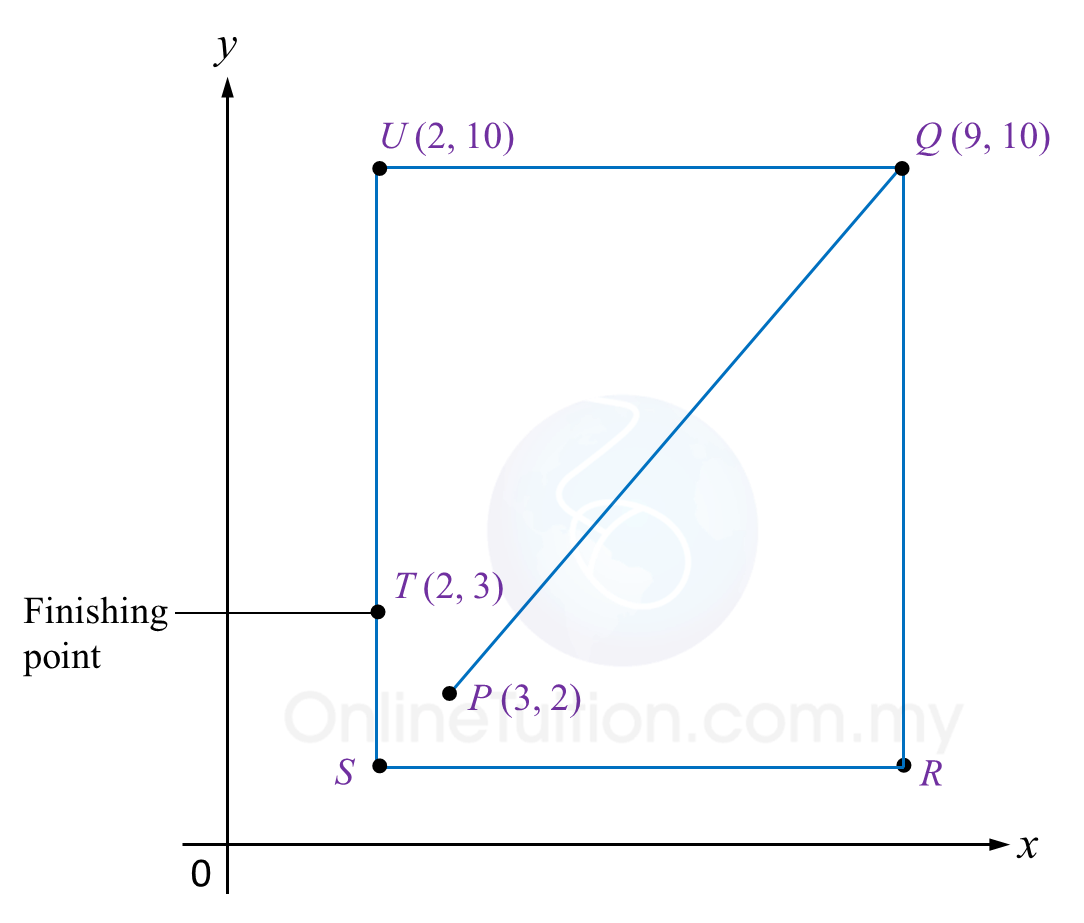The route of male participants is PQUT while the route for female participants is QRST. QR is parallel to UT whereas UQ is parallel to SR.
(a) Given the distance between point Q and point R is 9 km, state the coordinates of point R.
(b) It is given that the male participants start the run at point P and the female participants at point Q.
Find the difference of distance, in km, between the male and female participants.

Solution:
(a)
R = (9, 1)

(b)
Route of male participants
= PQ + QU + UT
= 10 + 7 + 7
= 24 km

Route of female participants
= QR + RS + ST
= 9 + 7 + 2
= 18 km

Difference in distance
= 24 – 18
= 6 km

Question 12:
Diagram below shows a parking lot in the shape of trapezium, RSTU. Two coordinates from the trapezium vertices are R(–30, –4) and S(20, –4).

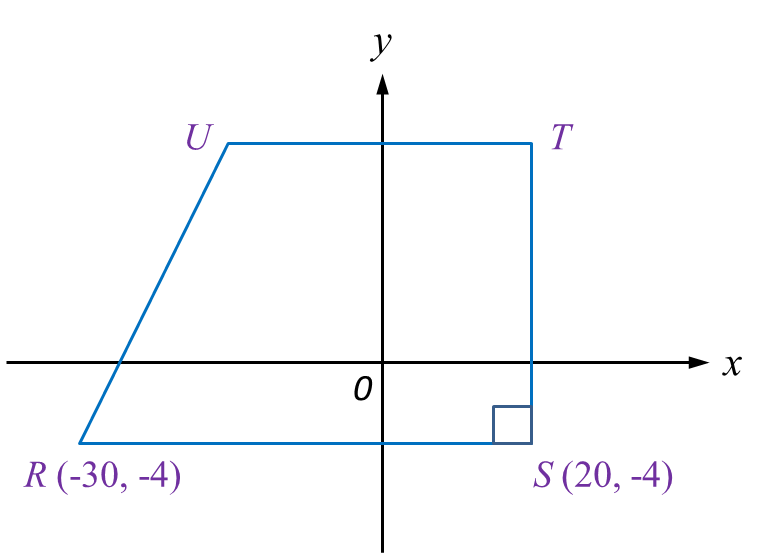
(a) Given the distance between vertex S and vertex T is 22 units.
State the coordinates of vertex T.
(b) Given the area of the parking lot is 946 unit², find the coordinates of U.

Solution:
(a)
y-coordinates of T = –4 + 22 = 18  
T = (20, 18)

(b)
$\begin{array}{l}\text{Area of trapezium}=946{\text{ unit}}^2\\ \frac{1}{2}\times \left(UT+RS\right)\times ST=946\\ \frac{1}{2}\times \left(UT+50\right)\times 22=946\\ UT+50=\frac{946\times 2}{22}\\ UT+50=86\\ UT=36\\ \\ x-\text{coordinate of point }U=20-36=-16\\ y-\text{coordinate of point }U=18\\ U=\left(-16,18\right)\end{array}$