2.2.1 Polygons II, PT3 Practice

Posted on December 17, 2017 by user

2.2.1 Polygons II, PT3 Practice

Question 1:

Diagram below shows a pentagon PQRST. TPU and RSV are straight lines.

Find the value of x.

Solution:

$\begin{array}{l} Sum of interior angles of a pentagon \\ = (5 - 2) \times 180^{o} \\ = 3 \times 180^{o} \\ = 540^{o} \\ ∠ T S R = 180^{o} - 70^{o} \\ = 110^{o} \\ ∠ T P Q = 180^{o} - 85^{o} \\ = 95^{o} \\ x^{o} = 540^{o} - (110^{o} + 105^{o} + 115^{o} + 95^{o}) \\ = 540^{o} - 425^{o} \\ = 115^{o} \\ x = 115 \end{array}$

Question 2:

In Diagram below, PQRSTU is a hexagon. APQ and BTS are straight lines.

Find the value of x + y.

Solution:

$\begin{array}{l} ∠ Q P U = 180^{o} - 160^{o} = 20^{o} \\ Reflex ∠ P U T = 360^{o} - 80^{o} = 280^{o} \\ ∠ U T S = 180^{o} - 120^{o} = 60^{o} \\ ∠ T S R = 180^{o} - 35^{o} = 145^{o} \\ Sum of interior angles of a hexagon \\ = (6 - 2) \times 180^{o} \\ = 720^{o} \\ x^{o} + y^{o} + 145^{o} + 60^{o} + 280^{o} + 20^{o} = 720^{o} \\ x^{o} + y^{o} = 720^{o} - 505^{o} \\ = 215^{o} \\ x + y = 215 \end{array}$

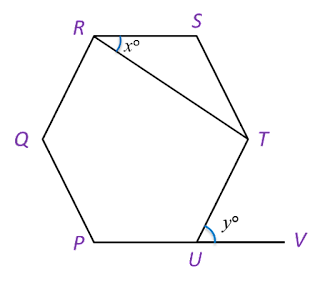
Question 3:

Diagram below shows a regular hexagon PQRSTU. PUV is a straight line.

Find the value of x + y.

Solution:

$\begin{array}{l} Size of each interior angle of a regular hexagon \\ = \frac{(6 - 2) \times 180^{o}}{6} \\ = 120^{o} \\ x^{o} = \frac{180^{o} - 120^{o}}{2} = 30^{o} \\ y^{o} = 180^{o} - 120^{o} \\ = 60^{o} \\ x^{o} + y^{o} = 30^{o} + 60^{o} \\ = 90^{o} \\ x + y = 90 \end{array}$

Question 4:

In the diagram below, KLMNP is a regular pentagon. LKS and MNQ are straight lines.

Find the value of x + y.

Solution:

$\begin{array}{l} Size of each interior angle of a regular pentagon \\ = \frac{(5 - 2) \times 180^{o}}{5} \\ = 108^{o} \\ ∠ P K S = ∠ P N Q = 180^{o} - 108^{o} = 72^{o} \\ Reflex angle ∠ K P N = 360^{o} - 108^{o} = 252^{o} \end{array}$

$\begin{array}{l} Sum of interior angles of a hexagon \\ = (6 - 2) \times 180^{o} \\ = 720^{o} \\ ∴ x^{o} + y^{o} + 72^{o} + 252^{o} + 72^{o} + 100^{o} = 720^{o} \\ x^{o} + y^{o} = 720^{o} - 496^{o} \\ = 224^{o} \\ x + y = 224 \end{array}$

Question 5:

In Diagram below, PQR is an isosceles triangle and PRU is a straight line.

Find the value of x + y.

Solution:

$\begin{array}{l} x^{o} = 180^{o} - 20^{o} - 20^{o} = 140^{o} \\ ∠ P R S = 180^{o} - 110^{o} = 70^{o} \\ y^{o} + 85^{o} + 75^{o} + 70^{o} = 360^{o} \\ y^{o} + 230^{o} = 360^{o} \\ y^{o} = 130^{o} \\ x^{o} + y^{o} = 140^{o} + 130^{o} \\ = 270^{o} \\ x + y = 270 \end{array}$