Home › 2018 › Page 2

Yearly Archives: 2018

14.2.1 Ratio, Rates and Proportions II, PT3 Focus Practice

Posted on by

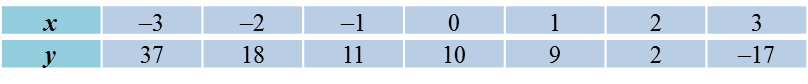
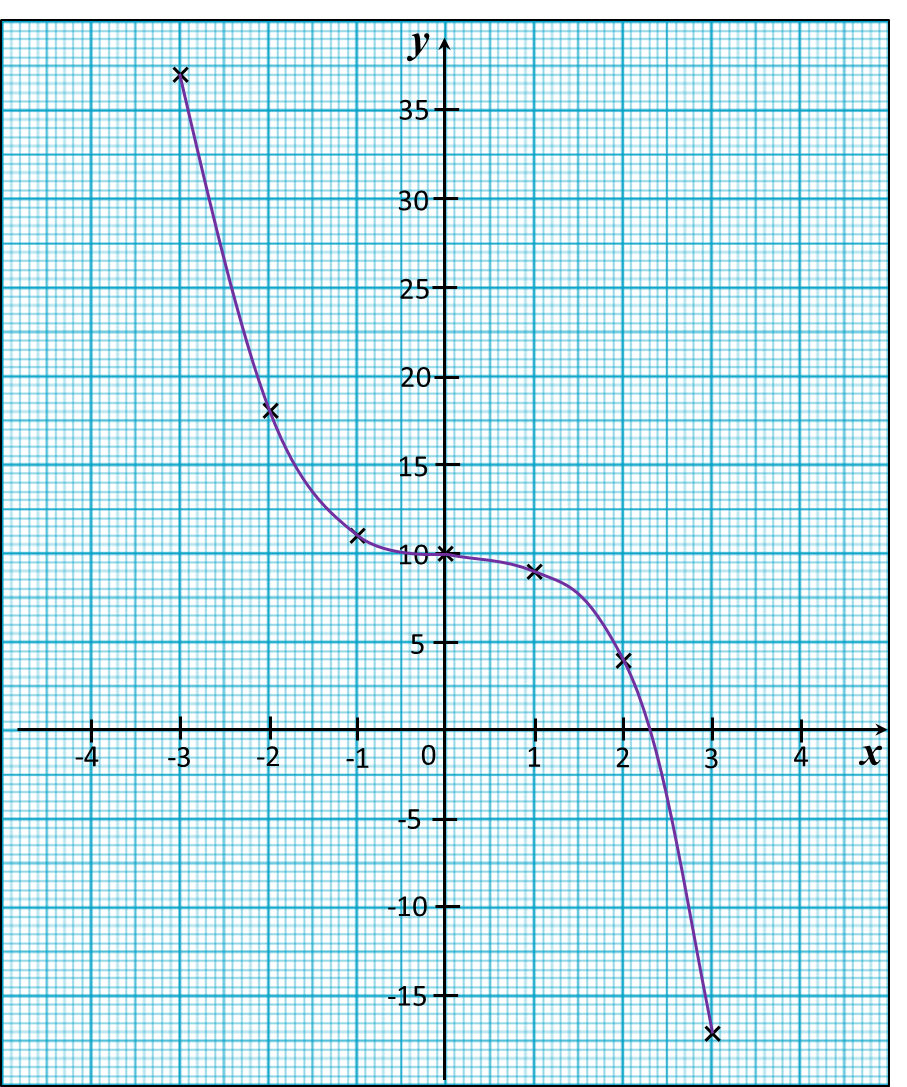
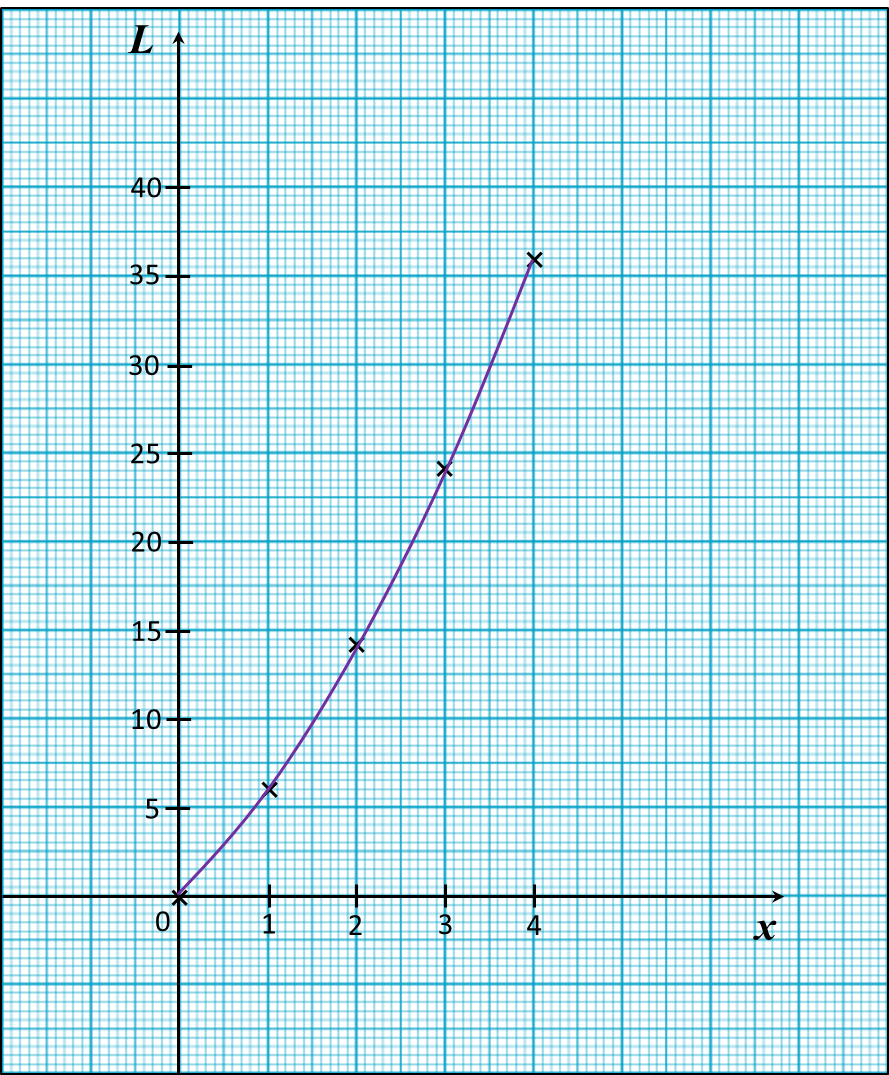
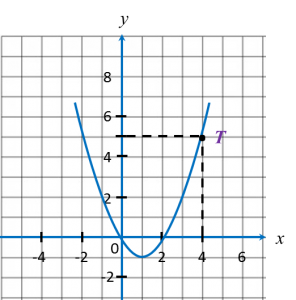
Question 1:
The distance from town A to town B is 120 km. A car leaves town A for town B at 11.00 a.m. The average speed is 80 km h-1 .
At what time does the car arrive at town B.

Solution:
Time taken=distance travelledaverage speed=120 km80 km h−1=32 hours= 1 hour 30 minutes1 hour 30 minutes after 11.00 a.m. is 12.30 p.m.


Question 2:
Kenny drives his car from town P to town Q at a distance of 180 km in 3 hours.
Faisal takes 30 minutes less than Kenny for the same journey.
Calculate the average speed, in km/h, of Faisal’s car.

Solution:
Time taken by Faisal= 3 hours−30 minutes= 3 hours−12 hour= 212 hoursAverage speed of Faisal's car=distance travelledtime taken=180 km212 hours=72 km/h


Question 3:
Rafidah drives her car from town L to town M at an average speed of 90 km/h for 2 hours 40 minutes. She continues her journey for a distance of 100 km from town M to town N and takes 1 hour 20 minutes.
Calculate the average speed, in km/h, for the journey from L to M.

Solution:
Distance=average speed×time takenDistance from L to M=90×24060  =90×223  =90×83  =240 kmTotal distance from L to N=240+100  =340 kmTotal time taken = 2 hours 40 minutes + 1 hour 20 minutes   = 4 hoursAverage speed for the journey from L to N=340 km4 h   =85 km/h


Question 4:
Susan drives at an average speed of 105 km/h from town F to town G.
The journey takes 3 hours.
Susan takes 30 minutes longer for her return journey from town G to town F. Calculate the average speed, in km/h, for the return journey.

Solution:
Distance from F to G= 105 km/h×3 hours=315 kmAverage speed for return journey=distance travelledtime taken=315 km312 hours=90 km/h


Question 5:
Table below shows the distances travelled and the average speeds for four vehicles.

Vehicle Distance (km) Average speed (km/h)
A 230 115
B 250 100
C 170 85
D 245 70
Which vehicles took the same time to complete the journey?

Solution:
Time taken for vehicle A=230115=2 hoursTime taken for vehicle B=250100=212 hoursTime taken for vehicle C=17085=2 hoursTime taken for vehicle D=24560=312 hours

Thus, the vehicles A and C took the same time to complete the journey.

13.2.3 Graphs of Functions, PT3 Focus Practice

Posted on by

Question 7:
Use graph paper to answer this question.
Table below shows the values of two variables, x and y, of a function.


The x-axis and the y-axis are provided on the graph paper on the answer space.
(a) By using a scale of 2 cm to 5 units, complete and label the y-axis.
(b) Based on the table above, plot the points on the graph paper.
(c) Hence, draw the graph of the function.

Answer:


Solution:



Question 8:
Use graph paper to answer this question.
Table below shows the values of two variables, x and y, of a function.


The x-axis and the y-axis are provided on the graph paper on the answer space.
(a) By using a scale of 2 cm to 2 units, complete and label the y-axis.
(b) Based on the table above, plot the points on the graph paper.
(c) Hence, draw the graph of the function.

Answer:


Solution:


13.2.2 Graphs of Functions, PT3 Focus Practice

Posted on by

13.2.2 Graphs of Functions, PT3 Focus Practice

Question 4:
Use graph paper to answer this question.
Table below shows the values of two variables, x and y, of a function.

x
–3
–2
–1
0
1
2
3
y
–19
–3
1
–1
–3
1
17
The x-axis and the y-axis are provided on the graph paper on the answer space.
(a) By using a scale of 2 cm to 5 units, complete and label the y-axis.
(b) Based on the table above, plot the points on the graph paper.
(c) Hence, draw the graph of the function.

Answer:



Solution:





Question 5:
Use graph paper to answer this question.
Table below shows the values of two variables, x and y, of a function.

x
–4
–3
–2
–1
0
1
2
y
31
17
7
1
–1
1
7
The x-axis and the y-axis are provided on the graph paper on the answer space.
(a) By using a scale of 2 cm to 5 units, complete and label the y-axis.
(b)   Based on the table above, plot the points on the graph paper.
(c) Hence, draw the graph of the function.


Answer:



Solution:







Question 6:
(a) Complete table below in the answer space for the equation L = x2 + 5x by writing the value of L when x = 2.
(b) Use graph paper to answer this part of the question. You may use a flexible curve rule.
By using a scale of 2 cm to 1 unit on the x-axis and 2 cm to 5 units on the y-axis, draw the graph of L = x2 + 5x for 0 ≤ x ≤ 4.

Answer:


Solution:
(a)
When x = 2
L = 22 + 5(2)
= 4 + 10
= 14  

(b)



13.2.1 Graphs of Functions, PT3 Focus Practice

Posted on by

13.2.1 Graphs of Functions, PT3 Focus Practice
 
Question 1:
 
In the figure shown, when x = 4, the value of y is

Solution:
 
T is the point on the graph where x = 4.
Hence, when x = 4, y = 5.


Question 2:
Diagram shows the graph of a function on a Cartesian plane.
 
The equation that represents the function is
 
Solution:
Use points (0, 6) and (-3, 0) to determine the function.

Function
Value of y when
x = 0
x = –3
y = x + 3
3
0
y = x – 3
–3
–6
y = 2x + 6
6
0 (correct)
y = 2x – 6
–6
–12

Question 3:
Table below shows the values of variables x and y of a function.
 
x
–2
0
1
y
–6
2
3
Which of the following functions is satisfied by the ordered pair?
A y = 7x + 2
B y = x3 + 2
C y = x + x2 – 2
D y = x2 + 2

Solution:
Only y = x3 + 2 is satisfied by all the ordered pairs.
(i) –6 = (–2)3 + 2
(ii)   2 = 03 + 2
(iii)  3 = 13 + 2
Answer = B

13.1 Graphs of Functions

Posted on by

13.1 Graphs of Functions
 
13.1.1 Functions
1.   Function is a relationship that expresses a variable (dependent) in terms of another variable (independent).
 
  

2.   y is a function of x if and only if every value of x corresponds to only one value of y.


13.1.2  Graphs of Functions
1.   A graph of function is a representation of the function as one or more lines on a coordinate plane.
Example:

2.   When drawing graphs of functions, it is best to observe these few points:
· choose suitable scales for both axes.
· use at least three points to draw a straight line to ensure accuracy.
· use at least five points to draw a curve to ensure accuracy.

12.2.2 Linear Inequalities, PT3 Focus Practice

Posted on by

Question 6:
List all the integer values of x which satisfy the following linear inequalities:
–2 < 3x + 1 ≤ 10

Solution:
–2 < 3x + 1
–3 < 3x
x > –1
x = 0, 1, 2, 3, …

3x + 1 ≤ 10
3x ≤ 9
x ≤ 3
x = 3, 2, 1, 0, …

Therefore x = 0, 1, 2, 3


Question 7:
List all the integer values of x which satisfy the following linear inequalities:
–5 < 2x – 3 ≤ 1

Solution:
–5 < 2x – 3
–5 + 3 < 2x
2x > –2
x > –1
x = 0, 1, 2, 3, …  

2x – 3 ≤ 1
2x ≤ 4
x ≤ 2
x = 2, 1, 0, –1, …

Therefore x = 0, 1, 2


Question 8:
Given that 3<√x−2<4 and x is an integer. List all the possible values of x.

Solution:
3<√x−2<432<x−2<429<x−2x>11 or   x−2<16x<1811<x<18x=12, 13, 14, 15, 16, 17


Question 9:
Find the biggest and the smallest integer of x that satisfy
3x + 2 ≥ –4 and 4 – x > 0.

Solution:
3x + 2 ≥ –4
3x ≥ –4 – 2
3x ≥ –6
x ≥ –2

4 – x > 0
–x > –4
x < 4

Smallest integer of x is –2, and the biggest integer of x is 3.



Question 10:
If x≤h≤y satisfy the two inequalities 7−h2≤5 and 3(h+2)≤20+h, find the values of x and y.

Solution:
7−h2≤5−h2≤5−7−h≤−4h≥43(h+2)≤20+h3h+6≤20+h2h≤14h≤74≤h≤7∴x=4,y=7

12.2.1 Linear Inequalities, PT3 Focus Practice

Posted on by

12.2.1 Linear Inequalities, PT3 Focus Practice

Question 1:
Draw a number line to represent the solution for the linear inequalities –3 < 5 – x  ≤ 4.
 
Solution:
–3 < 5 – x   and   5 – x  ≤ 4
x < 5 + 3   and   –x  ≤ 4 – 5
x < 8   and   –x ≤ –1 → x  ≥ 1

Thus, the solution is 1 ≤ x < 8

Question 2:
Solve the following simultaneous linear inequalities.
3x−5≤1    and    2−13x<3

Solution:
3x – 5 ≤ 1
3x  ≤ 1 + 5
3x  ≤ 6
x  ≤ 2

2−13x<36−x<9←  Multiply by 3  −x<9−6−x<3x>−3←  Multiply by −1  
The solution is –3 < x  ≤ 2.



Question 3:
The solution for the inequality 2 + x < 3x – 4 is
  
Solution:
2 + x < 3x – 4  
x – 3x < –4 – 2
–2x < –6
–x < –3
x > 3


Question 4:
The solution for the inequality –2 (6y + 3) < 3 (4 – 2y) is

Solution:
–2 (6y + 3) < 3 (4 – 2y)
–12y – 6 < 12 – 6y
–12y + 6y < 12 + 6
–6y < 18
–y < 3
y > –3 


Question 5:
Solve each of the following inequalities.
(a) 3x + 4 > 5x – 10
(b)   –3  ≤ 2x + 1 < 7

Solution:
(a)
3x + 4 > 5x – 10
3x – 5x > –10 – 4
–2x > –14
–x > –7
x < 7

(b)
–3 ≤ 2x + 1 < 7
–3 ≤ 2x + 1   and   2x + 1 < 7
–2x  ≤ 1 + 3   and   2x < 7 – 1
–2x  ≤ 4 and   2x < 6
x ≥ –2   and   x < 3

The solution is –2 ≤ x < 3.

12.1 Linear Inequalities

Posted on by

12.1 Linear Inequalities

12.1.1 Inequalities
1.   To write the relationship between two quantities which are not equal, we use the following inequality signs:
  > greater than
  < less than
  ≥ greater than or equal to
  ≤ less than or equal to

2.   7 > 4 also means 4 < 7.  7 > 4 and 4 < 7 are equivalent inequalities.


   12.1.2  Linear Inequalities in One Unknown
1.   An inequality in one unknown to the power of 1 is called a linear inequality.
Example: 2x + 5 > 7

2.   A linear inequality can be represented on a number line.
  Example:

12.1.3 Computation on Inequalities
1.   When a number is added or subtracted from both sides of an inequality, the condition of the inequality is unchanged.
Example:
Given 5 > 3
Then, 5 + 2 > 3 + 2 ← (symbol ‘>’ remains)
Hence, 7 > 5

2.   When both sides of an inequality are multiplied or divided by the same positive number, the condition of the inequality is unchanged.
Example:
Given 4x ≤ 16
Then, 4x ÷ 4 ≤ 16 ÷ 4 ← (symbol ‘≤’ remains)
Hence, x ≤ 4

3.   When both sides of an inequality are multiplied or divided by the same negative number, the inequality is reversed.
Example:
Given –3 > –5
Hence, 3 < 5
Given –5y > –10
Then, –5y ÷ 5 > –10 ÷ 5
 –y > –2
Hence, y < 2


12.1.4 Solve Inequalities in One Variable
To solve linear inequalities in one variable, use inverse operation to make the variable as the subject of the inequality.

Example:
Solve the following linear inequalities.
(a) 3−2x<1(b) 5−2x3≤7

Solution:
(a)
     3−2x<13−2x−3<1−3       −2x<−2           2x>2          2x2>22             x>1

(b)
        5−2x3≤7(5−2x3)×3≤7×3         5−2x≤21           −2x≤16               2x≥−16              2x2≥−162                 x≥−8



12.1.5 Simultaneous Linear Inequalities in One Variable
1.   The common values of two simultaneous inequalities are values which satisfy both linear inequalities.
The common values of the simultaneous linear inequalities x ≤ 3 and x > –1 is –1 < x ≤ 3.

2.   To solve two simultaneous linear inequalities is to find a single equivalent inequality which satisfies both inequalities.
 

6.3.2 Practising Critical and Analytical Thinking when Selecting Processed Food (Structured Questions)

Posted on by

Question 1:
Diagram 1 shows a labelled container of a processed food.



(a) Based on Diagram 1, identify two chemicals written on the label. [2 marks]

(b) Based on the label in Diagram 1, which chemical is used as a preservative? [1 mark]

(c) Name the method of food processing used in Diagram 1. [1 mark]

(d) What other information should be written on the label in Diagram 1 according to Food Regulations 1985?
Give two answers. [2 marks]


Answer:
(a)
1. Benzoic acid
2. Flavouring

(b)
Benzoic acid

(c)
Canning

(d)
- Name of manufacturer
- Address of manufacturer


11.2.2 Linear Equations II, PT3 Focus Practice

Posted on by

Question 6:
Diagram below shows 5 boxes of orange juice and 2 bottles of milk. Both orange juice and milk are mixed to produce 18 litres drink.


(a) Based on the above situation, write a linear equation.
(b) If 9 litres of drinks is produced by using 2 boxes of orange juice and 2 bottles of milk, find the volume, in litre, of orange juice in each box.

Solution:
Let j be the number of boxes of orange juice and m be the number of bottles of milk.
(a)
5j + 2m = 18

(b)
2j+2m=9  ............. (1)5j+2m=18 ............. (2)(2)−(1):3j=9j=93=33 litres of orange juice in each box.