Question 7:
Diagram below shows a curve y=14x2+3 which intersects the straight line y = x + 6 at point A.
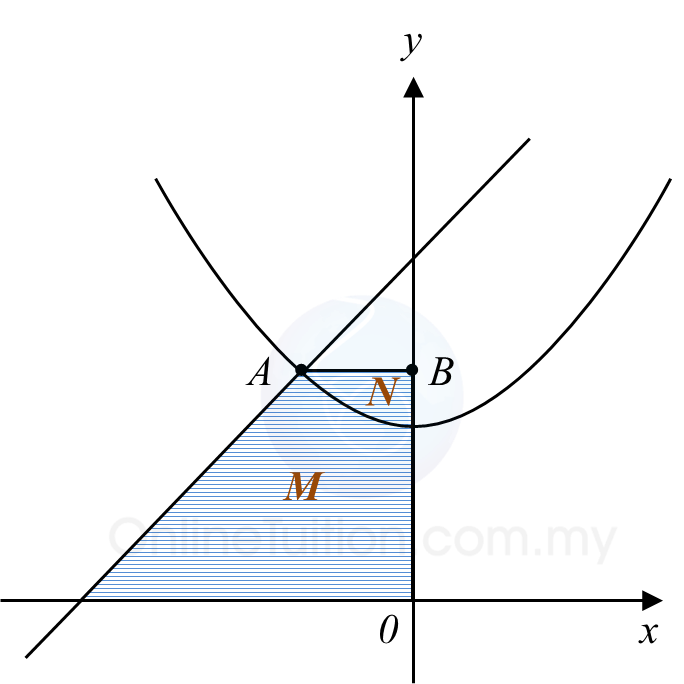
(a) Find the coordinates of A.
(b) Calculate
(i) the area of the shaded region M,
(ii) the volume generated, in terms of π, when the shaded region N is revolved 360o about the y-axis.
Solution:
(a)
y=14x2+3..........(1)y=x+6..........(2)Substitute (2) into (1),x+6=14x2+34x+24=x2+12x2−4x−12=0(x+2)(x−6)=0x=−2 or x=6 (rejected)When x=−2y=−2+6=4Therefore, A=(−2,4).
(b)(i)
At x-axis, y=0From y=x+6,x=−6Area of region M=Area of triangle+Area under the curve=12×(6−2)×4+∫0−2y dx=8+∫0−2(14x2+3) dx=8+[x34(3)+3x]0−2=8+[0−((−2)312+3(−2))]=8+[0−(−812−6)]=8+[0−(−203)]=1423 unit2
(b)(ii)
At y-axis, x=0, y=14(0)+3y=3y=14x2+34y=x2+12x2=4y−12Volume of N= π∫43x2dy= π∫43(4y−12)dy= π∫43(2y2−12y)dy=π[(2y2−12y)]43=π[(2(4)2−12(4))−(2(3)2−12(3))]=π(−16+18)=2π unit3
Diagram below shows a curve y=14x2+3 which intersects the straight line y = x + 6 at point A.

(a) Find the coordinates of A.
(b) Calculate
(i) the area of the shaded region M,
(ii) the volume generated, in terms of π, when the shaded region N is revolved 360o about the y-axis.
Solution:
(a)
y=14x2+3..........(1)y=x+6..........(2)Substitute (2) into (1),x+6=14x2+34x+24=x2+12x2−4x−12=0(x+2)(x−6)=0x=−2 or x=6 (rejected)When x=−2y=−2+6=4Therefore, A=(−2,4).
(b)(i)
At x-axis, y=0From y=x+6,x=−6Area of region M=Area of triangle+Area under the curve=12×(6−2)×4+∫0−2y dx=8+∫0−2(14x2+3) dx=8+[x34(3)+3x]0−2=8+[0−((−2)312+3(−2))]=8+[0−(−812−6)]=8+[0−(−203)]=1423 unit2
(b)(ii)
At y-axis, x=0, y=14(0)+3y=3y=14x2+34y=x2+12x2=4y−12Volume of N= π∫43x2dy= π∫43(4y−12)dy= π∫43(2y2−12y)dy=π[(2y2−12y)]43=π[(2(4)2−12(4))−(2(3)2−12(3))]=π(−16+18)=2π unit3