(Long Questions) – Question 7

Question 7:
Diagram below shows a quadrilateral ABCD where the sides AB and CD are parallel. ∠BAC is an obtuse angle.

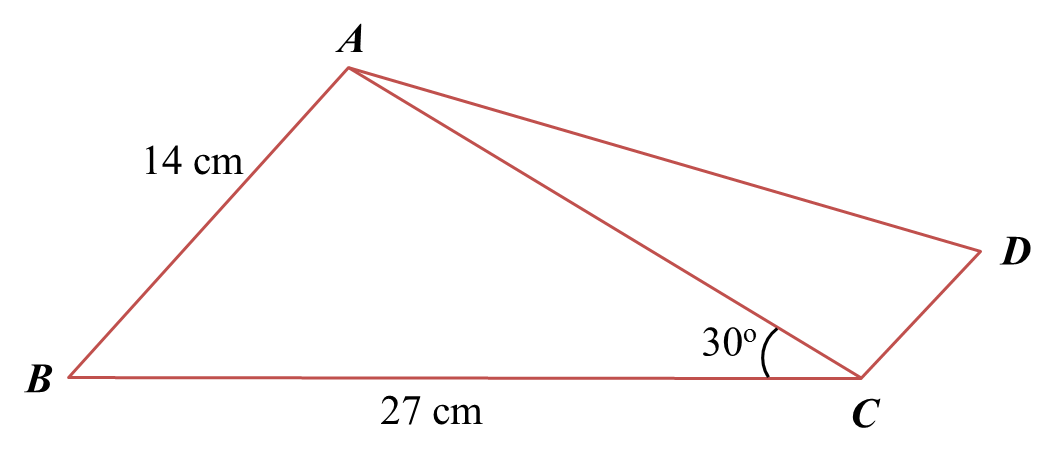
Given that AB = 14 cm, BC = 27 cm, ∠ACB = 30^o and AB : DC = 7 : 3.
Calculate
(a) ∠BAC.
(b) the length, in cm, of diagonal BD.
(c) the area, in cm², of quadrilateral ABCD.

Solution:
(a)
$\begin{array}{l}\frac{\sin \angle BAC}{27}=\frac{\sin {30}^o}{14}\\ \sin \angle BAC=\frac{\sin {30}^o}{14}\times 27\\ \sin \angle BAC=0.9643\\ \angle BAC={74.64}^o\\ \angle BAC\left(\text{obtuse}\right)={180}^o-{74.64}^o\\ ={105.36}^o\end{array}$


(b)
$\begin{array}{l}AB\text{ parallel with }DC\\ \angle BAC=\angle ACD\\ \angle BCD={105.36}^o+{30}^o\\ ={135.36}^o\\ \frac{DC}{AB}=\frac{3}{7}\\ DC=\frac{3}{7}\times 14\text{ cm}\\ =6\text{ cm}\\ \\ B{D}^2={27}^2+6^2-2\left(27\right)\left(6\right)\cos \angle BCD\\ B{D}^2=765-324\cos {135.36}^o\\ B{D}^2=995.54\\ BD=31.55\text{ cm}\end{array}$


(c)
$\begin{array}{l}\angle ABC={180}^o-{30}^o-{105.36}^o\\ ={44.64}^o\\ \\ A{C}^2={27}^2+{14}^2-2\left(27\right)\left(14\right)\cos \angle ABC\\ A{C}^2=925-756\cos {44.64}^o\\ A{C}^2=387.08\\ AC=19.67\text{ cm}\\ \\ \text{Area }△ABC\\ =\frac{1}{2}\left(14\right)\left(27\right)\sin {44.64}^o\\ =132.80{\text{ cm}}^2\\ \\ \text{Area }△ACD\\ =\frac{1}{2}\left(19.67\right)\left(6\right)\sin {105.36}^o\\ =56.90{\text{ cm}}^2\\ \\ \text{Area of quadrilateral }ABCD\\ =132.80+56.90\\ =189.7{\text{ cm}}^2\end{array}$