Long Questions (Question 7)

Posted on May 16, 2020 by Myhometuition

Question 7:

Diagram below shows a circle PQRT, centre O and radius 5 cm. AQB is a tangent to the circle at Q. The straight lines, AO and BO, intersect the circle at P and R respectively.

OPQR is a rhombus. ACB is an arc of a circle at centre O.

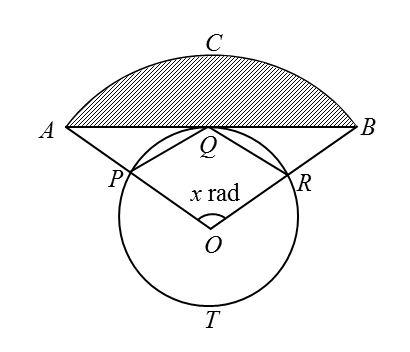Calculate

(a) the angle x , in terms of π ,

(b) the length , in cm , of the arc ACB ,

(c) the area, in cm²,of the shaded region.

Solution:

(a)

Rhombus has 4 equal sides, therefore OP = PQ = QR = OR = 5 cm

OR is radius to the circle, therefore OR = OQ = 5 cm

Triangles OQR and OQP are equilateral triangle,

Therefore, ∠ QOR= ∠QOP = 60^o

∠ POR = 120^o

x = 120^o × π/180^o

x = 2π/ 3 rad

(b)

cos ∠ AOQ= OQ / OA

cos 60^o = 5 / OA

OA = 10 cm

Length of arc, ACB,

s = r θ

Arc ACB = (10) (2π / 3)

Arc ACB = 20.94 cm

(c)

\begin{array}{l} Area of shaded region \\ = \frac{1}{2} r^{2} (θ - \sin θ) (change calculator to Rad mode) \\ = \frac{1}{2} {(10)}^{2} (\frac{2 π}{3} - \sin \frac{2 π}{3}) \\ = 50 (2.094 - 0.866) \\ = 61.40 {cm}^{2} \end{array}