Question 8:
Solution:
(a)
12AB=sin0.41×10→(Change the calculator to Rad mode)12AB=3.99∴
(b)
(c)

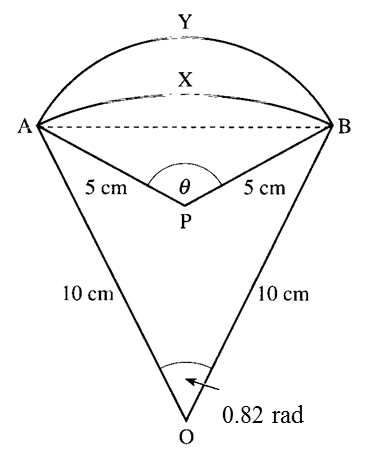
In the diagram above, AXB is an arc of a circle centre O and radius 10 cm with ∠AOB = 0.82 radian. AYB is an arc of a circle centre P and radius 5 cm with ∠APB = θ.
Calculate:
Calculate:
(a) the length of the chord AB,
(b) the value of θ in radians,
(c) the difference in length between the arcs AYB and AXB.
Solution:
(a)
12AB=sin0.41×10→(Change the calculator to Rad mode)12AB=3.99∴
(b)
(c)
Using s = rθ
Arcs AXB = 10 × 0.82 = 8.2 cm
Arcs AYB = 5 × 1.848 = 9.24 cm
Difference in length between the arcs AYB and AXB
= 9.24 – 8.2
= 1.04 cm