Question 6:
The masses of tomatoes in a farm are normally distributed with a mean of 130 g and standard deviation of 16 g. Tomato with weight more than 150 g is classified as grade ‘A’.
(a) A tomato is chosen at random from the farm. Find the probability that the tomato has a weight between 114 g and 150 g.
(b) It is found that 132 tomatoes in this farm are grade ‘A’. Find the total number of tomatoes in the farm.
Solution:
µ = 130
σ = 16
(a)
P(114<X<150)=P(114−13016<Z<150−13016)=P(−1<Z<1.25)=1−P(Z>1)−P(Z>1.25)=1−0.1587−0.1056=0.7357
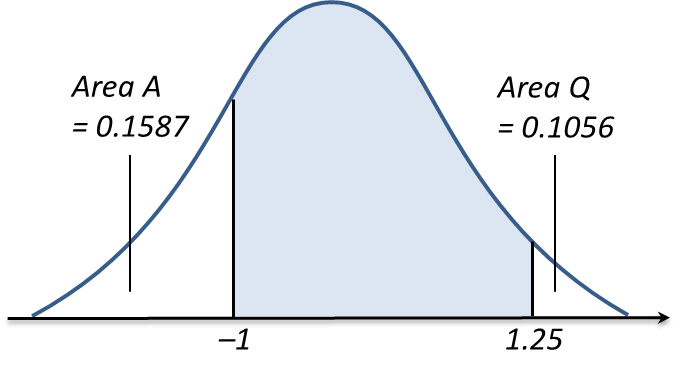

(b)
Probability of getting grade ‘A’ tomatoes,
P (X > 150) = P (Z > 1.25)
= 0.1056
Lets total number of tomatoes=N0.1056×N=132N=1320.1056N=1250