Question 5 (4 marks):
Diagram shows a circle with centre O.
 Diagram
Diagram
PR and QR are tangents to the circle at points P and Q respectively. It is given that the length of minor arc PQ is 4 cm and OR=5α cm.
Express in terms of α,
(a) the radius, r, of the circle,
(b) the area, A, of the shaded region.
Solution:
(a)
Given sPQ=4 rα=4 r=4α cm
(b)
PR=√(5α)2−(4α)2PR=√9α2PR=3αA= Area of shaded regionA= Area of quadrilateral OPRQ−Area of sector OPQ=2(Area of △ OPR)−12r2θ=2[12×3α×4α]−[12×(4α)2×α]=12α2−8α=12−8αα2 cm2
Diagram shows a circle with centre O.
 Diagram
Diagram PR and QR are tangents to the circle at points P and Q respectively. It is given that the length of minor arc PQ is 4 cm and OR=5α cm.
Express in terms of α,
(a) the radius, r, of the circle,
(b) the area, A, of the shaded region.
Solution:
(a)
Given sPQ=4 rα=4 r=4α cm
(b)
PR=√(5α)2−(4α)2PR=√9α2PR=3αA= Area of shaded regionA= Area of quadrilateral OPRQ−Area of sector OPQ=2(Area of △ OPR)−12r2θ=2[12×3α×4α]−[12×(4α)2×α]=12α2−8α=12−8αα2 cm2
Question 6 (3 marks):
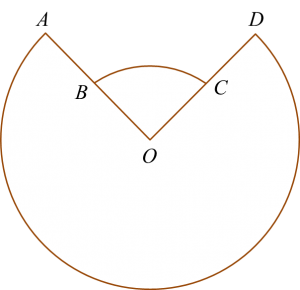
Diagram shows two sectors AOD and BOC of two concentric circles with centre O.
 Diagram
Diagram
The angle subtended at the centre O by the major arc AD is 7α radians and the perimeter of the whole diagram is 50 cm.
Given OB = r cm, OA = 2OB and ∠BOC = 2α, express r in terms of α.
Solution:
Length of major arc AOD=2r×7α=14rαLength of minor arc BOC=r×2α=2rαPerimeter of the whole diagram=50 cm14rα+2rα+r+r=5016rα+2r=508rα+r=25r(8α+1)=25r=258α+1
Diagram shows two sectors AOD and BOC of two concentric circles with centre O.
 Diagram
Diagram The angle subtended at the centre O by the major arc AD is 7α radians and the perimeter of the whole diagram is 50 cm.
Given OB = r cm, OA = 2OB and ∠BOC = 2α, express r in terms of α.
Solution:
Length of major arc AOD=2r×7α=14rαLength of minor arc BOC=r×2α=2rαPerimeter of the whole diagram=50 cm14rα+2rα+r+r=5016rα+2r=508rα+r=25r(8α+1)=25r=258α+1