Transformation III, Long Questions (Question 3)
Question 3:
(a) Transformation P is a reflection in the line x = m.
Transformation T is a translation
(4−2)
.
Transformation R is a clockwise rotation of 90o about the centre (0, 4).
(i) The point (6, 4) is the image of the point ( –2, 4) under the transformation P.
State the value of m.
(ii) Find the coordinates of the image of point (2, 8) under the following combined transformations:
(a) T2,
(b) TR.
(b) Diagram below shows trapezium CDFE and trapezium HEFG drawn on a Cartesian plane.
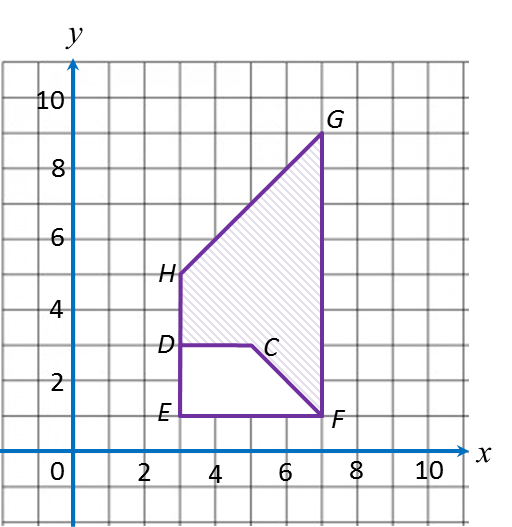
(i) HEFG is the image of CDEF under the combined transformation WU.
Describe in full the transformation:
(a) U (b) W
(ii) It is given that CDEF represents a region of area 60 m2.
Calculate the area, in m2, of the region represented by the shaded region.
Solution:
(a)(i)
(6,4)→P→(−2,4)m=6+(−2)2=2
(a)(ii)
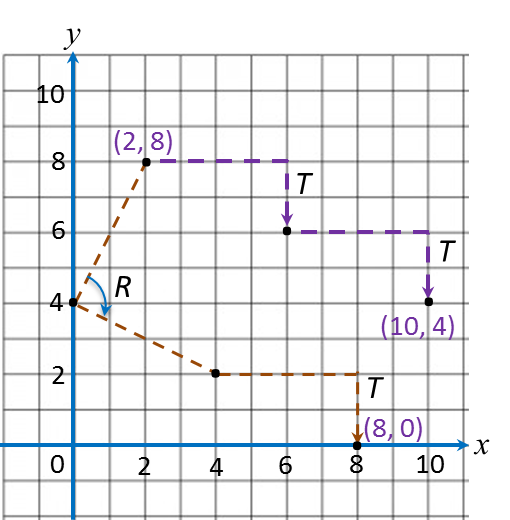
(a) (2, 8) → T → (6, 6) → T → (10, 4)
(b) (2, 8) → R → (4, 2) → T → (8, 0)
(b)(i)(a)
U: An anticlockwise rotation of 90oabout the centre A (3, 3).
(b)(i)(b)
Scale factor=HECD=42=2
W: An enlargement of scale factor 2 with centre B (3, 5).
W: An enlargement of scale factor 2 with centre B (3, 5).
(b)(ii)
Area of HEFG= (Scale factor)2 × Area of object
= 22 × area of CDEF
= 4 × 60
= 240 m2
Therefore,
Area of the shaded region
= Area of HEFG– area of CDEF
= 240 – 60
= 180 m2