5.7 SPM Practice (Long Questions 6)

Question 11 (5 marks):
Diagram 4 shows a parallelogram drawn on a Cartesian plane which represents the locations of Rahman's house, a cinema, a school and a shop.

It is given that the scale is 1 unit : 1 km.
(a) Calculate the distance, in km, between Rahman's house and the school.
(b) Find the equation of the straight line that links the school to the cinema.


Solution:
(a)
2y = 3x + 15
When y = 0
3x + 15 = 0
3x = –15
x = –5

Rahman's house = (–5, 0)
School = (3, 0)

Distance, between Rahman's house and the school
= 3 – (– 5)
= 8 units
= 8 km

(b)
$\begin{array}{l}2y=3x+15\\ y=\frac{3}{2}x+\frac{15}{2}\\ \text{Thus }m=\frac{3}{2}\\ \text{At point }\left(3,0\right),y_1=m{x}_1+c\\ 0=\frac{3}{2}\left(3\right)+c\\ \frac{9}{2}+c=0\\ c=-\frac{9}{2}\\ \\ \text{Thus, the linear equation is}\\ y=\frac{3}{2}x-\frac{9}{2}\\ 2y=3x-9\end{array}$

Question 12 (6 marks):
Diagram 6 shows two parallel straight lines, JK and LM, drawn on a Cartesian plane.
The straight line KM is parallel to the x-axis.

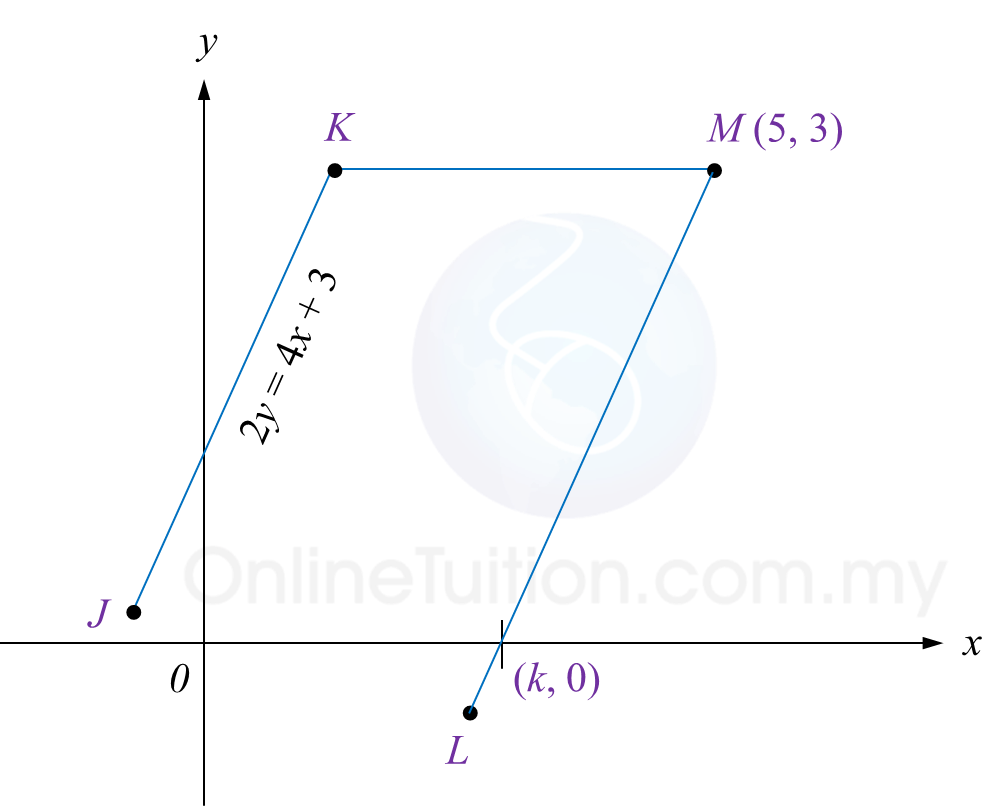Diagram 6

Find
(a) the equation of the straight line KM,
(b) the equation of the straight line LM,
(c) the value of k.



Solution:
(a)
The equation of the straight line KM is y = 3

(b)
$\begin{array}{l}\text{Given, equation of }JK:\\ 2y=4x+3\\ y=2x+\frac{3}{2}\\ \text{Thus, }{m}_{JK}=2\\ m_{LM}=m_{JK}=2\\ \\ y=mx+c\\ \text{At }M\left(5,3\right)\\ 3=2\left(5\right)+c\\ 3=10+c\\ c=-7\\ \\ \therefore \text{ Equation of the straight line }LM\\ \text{is }y=2x-7.\end{array}$


(c)
$\begin{array}{l}\text{Substitute }\left(k,0\right)\text{ into }y=2x-7\\ 0=2\left(k\right)-7\\ 7=2k\\ k=\frac{7}{2}\end{array}$