Short Question 5 – 8

Posted on April 23, 2020 by user

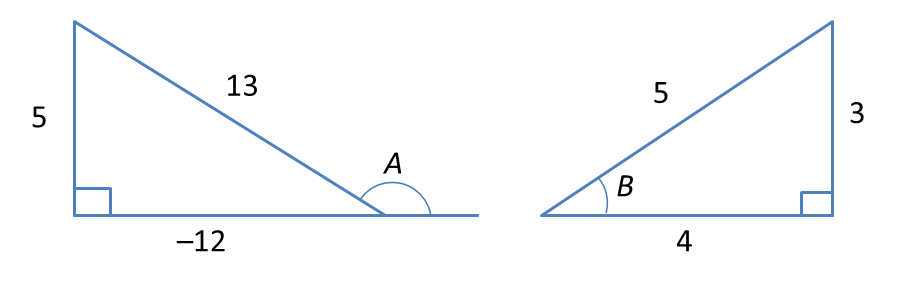
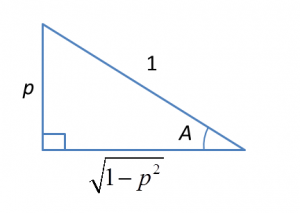
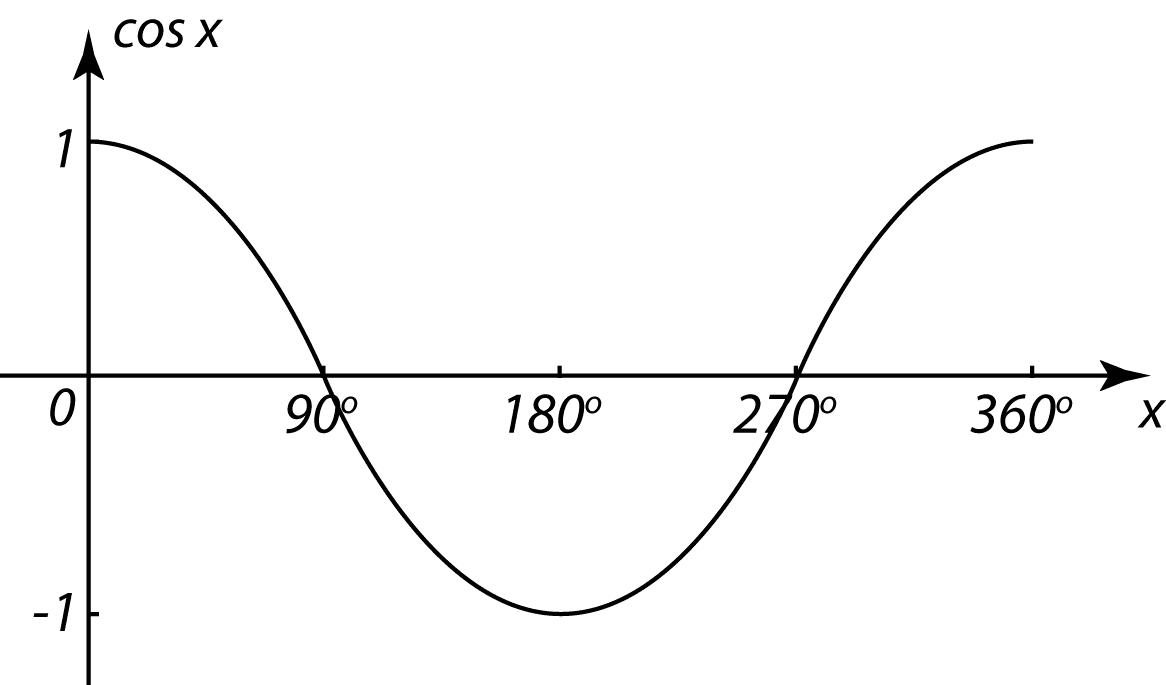
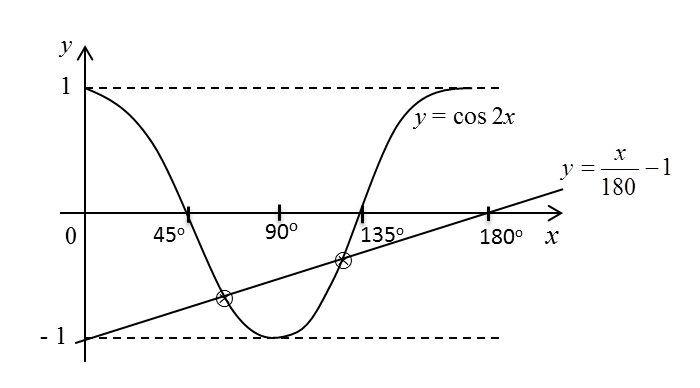
Question 4:
A committee that consists of 6 members is to be selected from 5 teachers and 4 students. Find the number of different committees that can be formed if
(a) there is no restriction,
(b) the number of teachers must exceed the number of students.

Solution:
(a)
Total number of committees = 5 + 4 = 9
6 members to be selected from 9 committees with no restriction

^{= 9} C_{6} = 84

(b)

\begin{array}{l} If the number of teachers must exceed the \\ number of students, the combination \\ = 4 teachers 2 students + 5 teachers 1 student \\ =^{5} C_{4} \times^{4} C_{2} +^{5} C_{5} \times^{4} C_{1} \\ = 30 + 4 \\ = 34 \end{array}

Question 5:
A school prefect committee that consists of 6 persons is to be chosen from 6 Malays, 5 Chinese and 4 Indians. Calculate the number of different committees that can be formed if the number of Malays, Chinese and Indians must be equal.

Solution:
Number of different committees that can be formed for 2 Malays, 2 Chinese and 2 Indians

\begin{array}{l} =^{6} C_{2} \times^{5} C_{2} \times^{4} C_{2} \\ = 900 \end{array}

Question 6:
There are 10 different flavour candies in a plastic bag.
Find
(a) the number of ways 3 candies can be chosen from the plastic bag.
(b) the number of ways at least 8 candies can be chosen from the plastic bag.

Solution:
(a)
Number of ways choosing 3 candies out of 10 candies

\begin{array}{l} =^{10} C_{3} \\ = 120 \end{array}

(b)
Number of ways choosing 8 candies =

=^{10} C_{8}

Number of ways choosing 9 candies =

^{10} C_{9}

Number of ways choosing 10 candies =

^{10} C_{10}

Hence, number of ways of choosing at least 8 candies

\begin{array}{l} =^{10} C_{8} +^{10} C_{9} +^{10} C_{10} \\ = 56 \end{array}

Short Question 1 – 3

Posted on April 22, 2020 by user

Question 1:

Solve the equation 3 cos 2A = 8 sin A – 5 for 0° ≤ A ≤ 360°.

Solution:

3 cos 2A = 8 sin A – 5

3(1–2 sin²A) = 8 sin A – 5

3 – 6 sin²A = 8 sin A – 5

6 sin²A + 8 sin A – 8 = 0

3 sin²A + 4 sin A – 4 = 0

(3 sin A – 2)(sin A + 2) = 0 ←( Factorise the equation )

3 sin A – 2 = 0

\sin A = \frac{2}{3} \leftarrow (sin A is positive in the 1st and 2nd quadrants .)

A = 41°49’, 138°11’

sin A + 2 = 0

sin A = –2 (no solution)

Hence A = 41°49’, 138°11’.

Question 2:

Solve the equation 2 cos 2x – cos x – 1 = 0 for 0° ≤ x ≤ 360°.

Solution:

2 cos 2x – cos x – 1 = 0

2 (2 cos² x – 1) – cos x – 1 = 0

4 cos² x– 2 – cos x – 1 = 0

4 cos² x– cos x – 3 = 0

(4 cos x + 3)(cos x – 1) = 0

cos x =

- \frac{3}{4}

basic angle = 41°24’

x = 138°36’, 221°24’

cos x = 1,

x = 0°, 360°

Hence x = 0°, 138°36’, 221°24’, 360°

Question 3:

Solve the equation 6 sec² x – 13 tan x = 0, 0° ≤ x ≤ 360°.

Solution:

6 sec² x – 13 tan x = 0

6 (1 + tan²x) – 13 tan x = 0
6 tan²x – 13 tan x + 6 = 0
(3 tan x – 2)(2 tan x – 3) = 0
tan x = 2/3 or tan x = 3/2

tan x = 2/3
Basic angle = 33.69°
x = 33.69°, 180° + 33.69°
x = 33.69°, 213.69°
Or
tan x = 3/2
Basic angle = 56.31°
x = 56.31°, 180° + 56.31°
x = 56.31°, 236.31°

Hence x = 33.69°, 56.31°, 213.69°, 236.31°.

Short Question 7 & 8

Posted on April 22, 2020 by user

Question 7:

It is given that

\sin A = \frac{5}{13} and \cos B = \frac{4}{5}

, where A is an obtuse angle and B is an acute angle.

Find

(a) tan A

(b) sin (A + B)

(c) cos (A – B)

Solution:

(a)

\tan A = - \frac{5}{12}

(b)

\begin{array}{l} \sin (A + B) = \sin A \cos B + \cos A \sin B \\ \sin (A + B) = (\frac{5}{13}) (\frac{4}{5}) + (- \frac{12}{13}) (\frac{3}{5}) \leftarrow \begin{array}{l} \cos A = - \frac{12}{13} \\ \sin B = \frac{3}{5} \end{array} \\ \sin (A + B) = \frac{4}{13} - \frac{36}{65} \\ \sin (A + B) = - \frac{16}{65} \end{array}

(c)

\begin{array}{l} \cos (A - B) = \cos A \cos B + \sin A \sin B \\ \cos (A - B) = (- \frac{12}{13}) (\frac{4}{5}) + (\frac{5}{13}) (\frac{3}{5}) \\ \cos (A - B) = - \frac{33}{65} \end{array}

Question 8:

If sin A = p, and 90° < A < 180°, express in terms of p

(a) tan A

(b) cos A

(c) sin 2A

Solution:

\begin{array}{l} Using Pythagoras Theorem, \\ Adjacent side \\ = \sqrt{1^{2} - p^{2}} \\ = \sqrt{1 - p^{2}} \end{array}

(a)

\tan A = - \frac{p}{\sqrt{1 - p^{2}}} \leftarrow \begin{array}{l} tan is negative at \\ second quadrant \end{array}

(b)

\cos A = - \sqrt{1 - p^{2}} \leftarrow \begin{array}{l} \cos is negative at \\ second quadrant \end{array}

(c)

\begin{array}{l} \sin A = 2 \sin A \cos A \\ \sin A = 2 (p) (- \sqrt{1 - p^{2}}) \\ \sin A = - 2 p \sqrt{1 - p^{2}} \end{array}

Short Question 11 – 14

Posted on April 22, 2020 by user

Question 11:

Prove the identity \frac{\cos^{2} x}{1 - \sin x} = 1 + \sin x

Solution:

\begin{array}{l} LHS \\ = \frac{\cos^{2} x}{1 - \sin x} \\ = \frac{1 - \sin^{2} x}{1 - \sin x} \leftarrow \sin^{2} x + \cos^{2} x = 1 \\ = \frac{(1 + \sin x) (1 - \sin x)}{1 - \sin x} \\ = 1 + \sin x \\ = RHS \\ ∴ Proven \end{array}

Question 12:

Prove the identity \sin^{2} x - \cos^{2} x = \frac{\tan^{2} x - 1}{\tan^{2} x + 1}

Solution:

\begin{array}{l} RHS \\ = \frac{\tan^{2} x - 1}{\tan^{2} x + 1} \\ = \frac{\frac{\sin^{2} x}{\cos^{2} x} - 1}{\frac{\sin^{2} x}{\cos^{2} x} + 1} \leftarrow \tan x = \frac{\sin x}{\cos x} \\ = \frac{\frac{\sin^{2} x - \cos^{2} x}{\cos^{2} x}}{\frac{\sin^{2} x + \cos^{2} x}{\cos^{2} x}} \\ = \frac{\sin^{2} x - \cos^{2} x}{\sin^{2} x + \cos^{2} x} \\ = \sin^{2} x - \cos^{2} x \leftarrow \sin^{2} x + \cos^{2} x = 1 \\ = LHS \\ ∴ Proven \end{array}

Question 13:

Prove the identity \tan^{2} θ - \sin^{2} θ = \tan^{2} θ \sin^{2} θ

Solution:

\begin{array}{l} LHS \\ = \tan^{2} θ - \sin^{2} θ \\ = \frac{\sin^{2} θ}{\cos^{2} θ} - \sin^{2} θ \\ = \frac{\sin^{2} θ - \sin^{2} θ \cos^{2} θ}{\cos^{2} θ} \\ = \frac{\sin^{2} θ (1 - \cos^{2} θ)}{\cos^{2} θ} \\ = \frac{\sin^{2} θ \sin^{2} θ}{\cos^{2} θ} \\ = (\frac{\sin^{2} θ}{\cos^{2} θ}) (\sin^{2} θ) \\ = \tan^{2} θ \sin^{2} θ \\ = RHS \\ ∴ Proven \end{array}

Question 14:

{Prove the identity cosec}^{2} θ (\sec^{2} θ - \tan^{2} θ) - 1 = \cot^{2} θ

Solution:

\begin{array}{l} LHS \\ = {cosec}^{2} θ (\sec^{2} θ - \tan^{2} θ) - 1 \\ = {cosec}^{2} θ (1) - 1 \leftarrow \begin{array}{l} \tan^{2} θ + 1 = \sec^{2} θ \\ \sec^{2} θ - \tan^{2} θ = 1 \end{array} \\ = {cosec}^{2} θ - 1 \\ = \cot^{2} θ \leftarrow \begin{array}{l} 1 + \cot^{2} θ = \cos e c^{2} θ \\ \cos e c^{2} θ - 1 = \cot^{2} θ \end{array} \\ = RHS \\ ∴ Proven \end{array}

5.6b Solving Trigonometric Equation (Factorization)

Posted on April 22, 2020 by user

5.6b Solving Trigonometric Equation (Factorization)

Example:

Find all the angles that satisfy each of the following equations for 0° £ x £ 360°.

(a)

\cot x = - 2 \cos x

(b)

3 \sec x = 4 \cos x

(c)

16 \tan x = \cot x

Solution:
(a)

\begin{array}{l} \cot x = - 2 \cos x \\ \frac{\cos x}{\sin x} = - 2 \cos x \\ \cos x = - 2 \cos x \sin x \\ \cos x + 2 \sin x \cos x = 0 \\ \cos x (1 + 2 \sin x) = 0 \\ \cos x = 0 \\ x = 90^{\circ}, 270^{\circ} \\ 1 + 2 \sin x = 0 \\ \sin x = - \frac{1}{2} \\ Basic ∠ = 3 0^{\circ} \\ x = (180^{\circ} + 30^{\circ}), (360^{\circ} - 30^{\circ}) \\ x = 210^{\circ}, 330^{\circ} \\ ∴ x = 90^{\circ}, 210^{\circ}, 270^{\circ}, 330^{\circ} \end{array}

(b)

\begin{array}{l} 3 \sec x = 4 \cos x \\ \frac{3}{\cos x} = 4 \cos x \\ 3 = 4 \cos^{2} x \\ \cos^{2} x = \frac{3}{4} \\ \cos x = \pm \frac{\sqrt{3}}{2} \\ Basic ∠ = 30^{\circ} \\ x = 30^{\circ}, (180^{\circ} - 30^{\circ}), (180^{\circ} + 30^{\circ}), (360^{\circ} - 30^{\circ}) \\ x = 30^{\circ}, 150^{\circ}, 210^{\circ}, 330^{\circ} \end{array}

(c)

\begin{array}{l} 16 \tan x = \cot x \\ 16 \tan x = \frac{1}{\tan x} \\ \tan^{2} x = \frac{1}{16} \\ \tan x = \pm \frac{1}{4} \\ Basic ∠ = {14.04}^{\circ} \\ x = {14.04}^{\circ}, (180^{\circ} - {14.04}^{\circ}), \\ (180^{\circ} + {14.04}^{\circ}), (360^{\circ} - {14.04}^{\circ}) \\ x = {14.04}^{\circ}, {165.96}^{\circ}, {194.04}^{\circ}, {345.96}^{\circ} \end{array}

5.6c Solving Trigonometric Equation (Form Quadratic Equation In Sinx/ Cosx/ Tanx/ Cosecx/ Secx/ Cotx)

Posted on April 22, 2020 by user

(C) Solving Trigonometric Equation (Form Quadratic Equation in sinx/ cosx/ tanx/ cosecx/ secx/ cotx)

Example:
Find all the angles between 0° and 360° that satisfy each of the following equations.
(a) 3 sin² x – 2 sin x – 1 = 0
(b) 2 sin x = cosec x + 1
(c) 5 sin² x = 2 (1 + cos x)
(d) 2 sec x = 1 + cos x
(e) 2 cot² x + 8 = 7 cosec x

Solution:
(a)
3 sin² x – 2 sin x – 1 = 0
(3 sin x + 1)(sin x – 1) = 0
sin x = –⅓, sin x = 1
sin x = –⅓
basic angle = 19.47°
x = 180° + 19.47°, 360° – 19.47°
x = 199.47°, 340.53
sin x = 1, x = 90°
Hence x = 90°, 199.47°, 340.53°

(b)
2 sin x = cosec x + 1

2 \sin x = \frac{1}{\sin x} + 1

2 sin ² x = 1 + sin x
2 sin ² x – sin x – 1 =0
(2 sin x + 1)(sin x – 1) = 0
sin x = –½, sin x = 1
sin x = –½
basic angle = 30°
x = 180° + 30°, 360° – 30°
x = 210°, 330°
sin x = 1, x = 90°
Hence x = 90°, 210°, 330°

(c)
5 sin² x = 2 (1 + cos x)
5 (1 – cos² x) = 2 + 2 cos x
5 – 5 cos² x – 2 – 2 cos x = 0
– 5 cos² x – 2 cos x + 3 = 0
5 cos² x + 2 cos x – 3 = 0
(5 cos x – 3)(cos x + 1) = 0

\begin{array}{l} \cos x = \frac{3}{5}, \cos x = - 1 \\ \cos x = \frac{3}{5} \end{array}

basic angle = 53.13°
x = 53.13°, 360° – 53.13°
x = 53.13°, 306.87°
cos x = – 1
x = 180°
Hence x = 53.13°, 180°, 306.87°

(d)
2 sec x = 1 + cos x

\frac{2}{\cos x} = 1 + \cos x

2 = cos x + cos² x

cos² x + cos x – 2 = 0

(cos x – 1)(cos x + 2) = 0

cos x = 1

x = 0°, 360°

cos x = –2 (not accepted)

Hence x = 0°, 360°

(e)
2 cot² x + 8 = 7 cosec x
2 (cosec² x – 1) + 8 = 7 cosec x
2 cosec² x – 2 – 7 cosec x + 8 = 0

2 cosec²x – 7 cosec x + 6 = 0

(2 cosec x – 3)(cosec x – 2) = 0

\begin{array}{l} \cos e c x = \frac{3}{2}, \cos e c x = 2 \\ \sin x = \frac{2}{3}, \sin x = \frac{1}{2} \\ \sin x = \frac{2}{3} \\ basic angle = {41.81}^{\circ} \\ x = {41.81}^{\circ}, 180 - {41.81}^{\circ} \\ \sin x = \frac{1}{2} \\ {basic angle = 30}^{\circ} \\ x = 30^{\circ}, 180 - 30^{\circ} \\ Hence x = 30^{\circ}, {41.81}^{\circ}, {138.19}^{\circ}, 150^{\circ} \end{array}

5.5.1 Proving Trigonometric Identities Using Addition Formula And Double Angle Formulae (Part 1)

Posted on April 22, 2020 by user

Example 2:

Prove each of the following trigonometric identities.

\begin{array}{l} (a) \frac{1 + \cos 2 x}{\sin 2 x} = \cot x \\ (b) \cot A \sec 2 A = \cot A + \tan 2 A \\ (c) \frac{s i n x}{1 - c o s x} = \cot \frac{x}{2} \end{array}

Solution:

(a)

\begin{array}{l} L H S \\ = \frac{1 + \cos 2 x}{\sin 2 x} \\ = \frac{1 + (2 \cos^{2} x - 1)}{2 \sin x \cos x} \\ = \frac{2 \cos^{2} x}{2 \sin x \cos x} \\ = \frac{\cos x}{\sin x} \\ = \cot x = R H S (proven) \end{array}

(b)

\begin{array}{l} R H S \\ = \cot A + \tan 2 A \\ = \frac{\cos A}{\sin A} + \frac{\sin 2 A}{\cos 2 A} \\ = \frac{\cos A \cos 2 A + \sin A \sin 2 A}{\sin A \cos 2 A} \\ = \frac{\cos A (\cos^{2} A - \sin^{2} A) + \sin A (2 \sin A \cos A)}{\sin A \cos 2 A} \\ = \frac{\cos^{3} A - \cos A \sin^{2} A + 2 \sin^{2} A \cos A}{\sin A \cos 2 A} \\ = \frac{\cos^{3} A + \cos A \sin^{2} A}{\sin A \cos 2 A} \\ = \frac{\cos A (\cos^{2} A + \sin^{2} A)}{\sin A \cos 2 A} \\ = \frac{\cos A}{\sin A \cos 2 A} \leftarrow \sin^{2} A + \cos^{2} A = 1 \\ = (\frac{\cos A}{\sin A}) (\frac{1}{\cos 2 A}) \\ = \cot A \sec 2 A \end{array}

(c)

\begin{array}{l} L H S \\ = \frac{s i n x}{1 - c o s x} \\ = \frac{2 s i n \frac{x}{2} \cos \frac{x}{2}}{1 - (1 - 2 s i n^{2} \frac{x}{2})} \leftarrow \begin{array}{l} \sin x = 2 s i n \frac{x}{2} \cos \frac{x}{2}, \\ \cos x = 1 - 2 \sin^{2} \frac{x}{2} \end{array} \\ = \frac{2 s i n \frac{x}{2} \cos \frac{x}{2}}{2 s i n^{2} \frac{x}{2}} = \frac{\cos \frac{x}{2}}{s i n \frac{x}{2}} \\ = \cot \frac{x}{2} = R H S (proven) \end{array}

5.6d Solving Trigonometric Equations (Involving Addition Formulae And Double Angle Formulae)

Posted on April 22, 2020 by user

(D) Solving Trigonometric Equations (Involving Addition Formulae and Double Angle Formulae)

Example 1 (Addition Formulae):
Solve the following equation for 0^o ≤ x ≤ 360^o:

(a) sin ( x – 25^o) = 3 sin ( x + 25^o)

(b) 3 cos ( 2x + 10^o) = 2

Solution:

(a)
sin ( x – 25^o) = 3 sin ( x + 25^o)

sin x cos 25^o – cos x sin 25^o = 3 (sin x cos 25^o + cos x sin 25^o)

sin x cos 25^o – cos x sin 25^o = 3 sin x cos 25^o + 3 cos x sin 25^o

– sin x cos 25^o = 4 cos x sin 25^o

\frac{\sin x}{\cos x} = - \frac{4 \sin 25^{\circ}}{2 \cos 25^{\circ}}

tan x = – 2 tan 25^o

tan x = – 2 (0.4663)

tan x = – 0.9326

basic angle x = 43^o

The reference angles of x = 43^o are in the second and fourth quadrants.

Hence, x = 180^o– 43^o, 360^o– 43^o

x = 137^o, 317^o

(b)

3 cos ( 2x + 10^o) = 2 ← (Take the angles in the range of 0^o ≤ x ≤ 720^o, which in 2 complete revolutions)

cos ( 2x + 10^o) = ⅔

basic angle ( 2x + 10^o) = 48.19^o

2x + 10^o = 48.19^o, 360^o– 48.19^o, 360^o+ 48.19^o, 720^o– 48.19^o

2x + 10^o = 48.19^o, 311.81^o, 408.19^o, 671.81^o

2x = 38.19^o, 301.81^o, 398.19^o, 661.81^o

x = 19.10^o, 150.91^o, 199.10^o, 330.91^o

Example 2 (Double Angle):

Find all the angles that satisfy the equation 5 cos 2A + 9 sin A = 7,
0° < A < 360°.

Solution:

5 cos 2A + 9 sin A = 7

5 (1 – 2 sin²A) + 9 sin A = 7 ← (substitution of cos 2A = 1 – 2 sin²A is used. Then the whole equation will be in terms of sin A)

5 – 10 sin²A + 9 sin A – 7 = 0

– 10 sin²A + 9 sin A – 2 = 0

10 sin²A – 9 sin A + 2 = 0

(2 sin A – 1)(5 sin A – 2) = 0 ← (Factorise)

sin A = ½ = 0.5 or sin A=

\frac{2}{5}

= 0.4

When sin A = 0.5,

Basic angle = 30º

A = 30º, 180º – 30º

A = 30º, 150º

When sin A = 0.4,

Basic angle = 23.58º

A = 23.58º, 180º – 23.58º

A = 23.58º, 156.42º

Hence A = 23.58º, 30º, 150º, 156.42º.

Example 3 (Double Angle):

Find all the possible values of θ between 0 and 2π rad for the equation sin 2θ = sin θ

Solution:

sin 2θ = sin θ

2 sin θ cos θ = sin θ ← (sin 2θ = 2 sin θ cos θ)

2 sin θ cos θ – sin θ = 0

sin θ (2 cos θ – 1) = 0 ← (Factorise)

sin θ = 0 or 2 cos θ – 1 = 0

When sin θ = 0

θ = 0, π, 2π

When 2 cos θ – 1= 0

cos θ = ½

\begin{array}{l} θ = \frac{1}{3} π, \frac{5}{3} π \\ Hence, θ = 0, \frac{1}{3} π, π, \frac{5}{3} π, 2 π . \end{array}

5.2.2 Six Trigonometric Functions of Any Angle

Posted on April 22, 2020 by user

5.2b Six Trigonometric Functions of Any Angle

(B) Special Angles

(1) Value of Special Angle 30° and 60°

\begin{array}{l} (a) \sin 30^{o} = \frac{1}{2} (b) c o s 30^{o} = \frac{\sqrt{3}}{2} (c) t a n 30^{o} = \frac{1}{\sqrt{3}} \\ (d) \sin 60^{o} = \frac{\sqrt{3}}{2} (e) c o s 60^{o} = \frac{1}{2} (f) t a n 60^{o} = \sqrt{3} \end{array}

(2) Value of Special Angle 45°

(a) \sin 45^{o} = \frac{1}{\sqrt{2}} (b) c o s 45^{o} = \frac{1}{\sqrt{2}} (c) t a n 45^{o} = 1

(3) Value of Special Angle 0°, 90°, 180°, 270°, 360°

(a) y = sin x

x	0^o	90^o	180^o	270^o	360^o
sin	0	1	0	-1	0

(b) y = cos x

(c) y = tan x

x	0^o	90^o	180^o	270^o	360^o
tan	0	∞	0	∞	0

Long Question 1 & 2

Posted on April 22, 2020 by user

Question 1:

(a) Sketch the graph of y = cos 2x for 0° ≤ x ≤ 180°.

(b) Hence, by drawing a suitable straight line on the same axes, find the number of solutions satisfying the equation

2 {sin}^{2} x = 2 - \frac{x}{180}

for 0° ≤ x ≤ 180°.

Solution:
(a)(b)

\begin{array}{l} 2 {sin}^{2} x = 2 - \frac{x}{180} \\ 1 - 2 {sin}^{2} x = 1 - (2 - \frac{x}{180}) \\ \cos 2 x = \frac{x}{180} - 1 \\ y = \frac{x}{180} - 1 \\ x = 0, y = - 1 \\ x = 180, y = 0 \\ Number of solutions = 2 \end{array}

Question 2:
(a) Sketch the graph of

y = \frac{3}{2} \cos 2 x for 0 \leq x \leq \frac{3}{2} π .

(b) Hence, using the same axes, sketch a suitable straight line to find the number of solutions to the equation

\frac{4}{3 π} x - \cos 2 x = \frac{3}{2} for 0 \leq x \leq \frac{3}{2} π

State the number of solutions.

Solution:
(a)(b)

\begin{array}{l} \frac{4}{3 π} x - \cos 2 x = \frac{3}{2} \\ \cos 2 x = \frac{4}{3 π} x - \frac{3}{2} \\ \frac{3}{2} \cos 2 x = \frac{3}{2} (\frac{4}{3 π} x - \frac{3}{2}) \\ y = \frac{2}{π} x - \frac{9}{4} \\ To sketch the graph of y = \frac{2}{π} x - \frac{9}{4} \\ x = 0, y = - \frac{9}{4} \\ x = \frac{3 π}{2}, y = \frac{3}{4} \\ Number of solutions \\ = Number of intersection points \\ = 3 \end{array}