Cotx)

Posted on April 22, 2020 by user

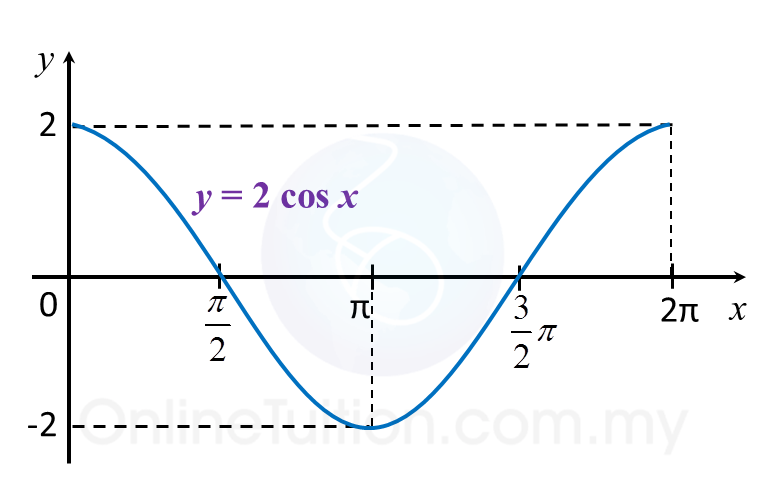
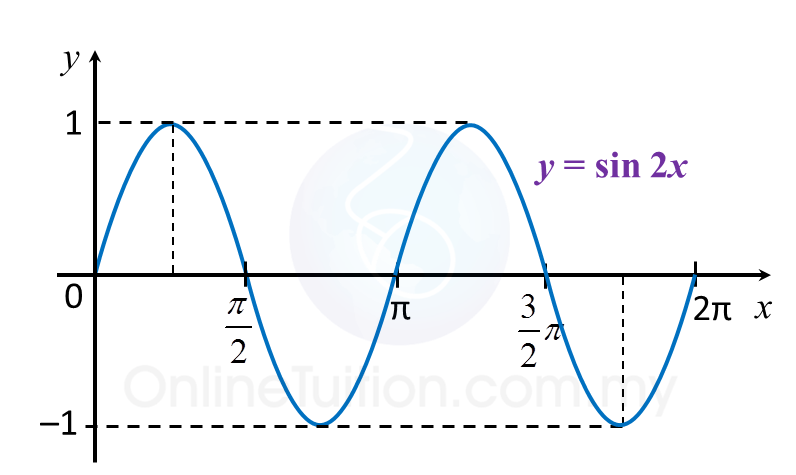
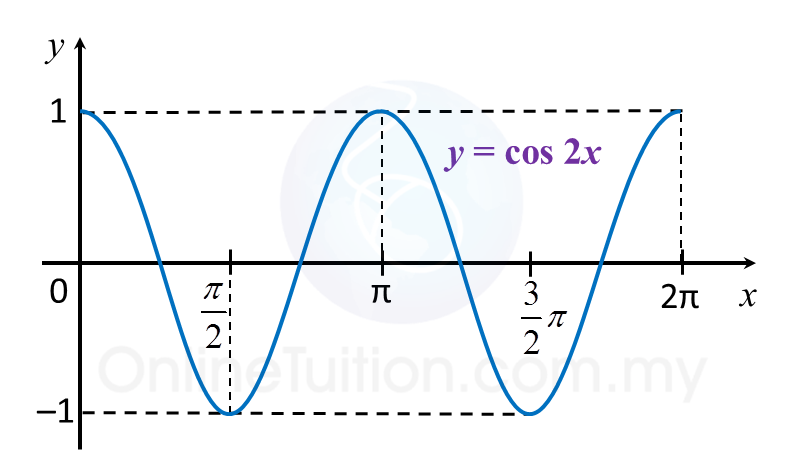
5.6 Simple Trigonometric Equations

Steps to solve simple trigonometric equation:
(1) Determine the range of values of the required angles.
(2) Find a basic angle by using calculator.
(3) Determine the quadrants the angle should be.
(4) Determine the values of angles in those quadrants.

(A) Solving Trigonometric Equation (Basic Equation in sinx/ cosx/ tanx/ cosecx/ secx/ cotx)

Example:
Find all the values of θ for 0° < θ < 360° that satisfy each of the following trigonometric equations.
(a) sin θ = 0.6137
(b) cos θ = 0.2377
(c) tan θ = 2.7825
(d) sin θ = -0.8537

(e) sin 2θ = 0.5293

Solution:

(a)
sin θ = 0.6137

basic angle = sinˉ¹ 0.6137 = 37.86°

θ = 37.86°, 180°-37.86°

θ = 37.86°, 142.14°

(b)
cos θ = 0.2377

basic angle = cosˉ¹ 0.2377 = 76.25°

θ = 76.25°, 360° – 76.25°

θ = 76.25°, 283.75°

(c)
tan θ = 2.7825

basic angle = tanˉ¹ 2.7825 = 70.23°

θ = 70.23°, 180° + 70.23°

θ = 70.23°, 250.23°

(d)
sin θ = -0.8537

basic angle = sinˉ¹ 0.8537 = 58.62°

θ = 180° + 58.62°, 360° – 58.62°

θ = 238.62°, 301.38°

(e)
sin 2θ = 0.5293

basic angle = 31.96°

0° < θ < 360°

0° < 2θ < 720°

2θ = 31.96°, 180° – 31.96°, 360° + 31.96°, 360° + 180° – 31.96°

2θ = 31.96°, 148.04°, 391.96°, 508.04°

θ = 15.98°, 74.02°, 195.98°, 254.02°

Short Question 9 & 10

Posted on April 22, 2020 by user

Question 9:

Given that sin θ =

\frac{3}{5}

, where θ is an acute angle, without using tables or a calculator, find the values of

(a) sin (180º + θ),

(b) cos (180º – θ),

(c) tan (360º + θ).

Solution:
(a)

\sin θ = \frac{3}{5} \cos θ = \frac{4}{5} \tan θ = \frac{3}{4}

sin (180º + θ)

= sin 180º cos θ + cos 180º sin θ

= (0) cos θ + (– 1) sin θ

= – sin θ

- \frac{3}{5}

(b)

cos (180º – θ)

= cos 180º cos θ + sin 180º sin θ

= (– 1) cos θ + (0) sin θ

= – cos θ

- \frac{4}{5}

(c)

\begin{array}{l} \tan (360^{\circ} + θ) \\ = \frac{\tan 360^{\circ} + \tan θ}{1 - \tan 360^{\circ} \tan θ} \\ = \frac{0 + \tan θ}{1 - (0) (\tan θ)} \\ = \tan θ \\ = \frac{3}{4} \end{array}

Question 10:

Prove each of the following trigonometric identities.

(a) cot²x – cot² x cos²x = cos² x

(b) \frac{\sec x}{\sec x - \cos x} = \cos e c^{2} x

Solution:

(a)

\begin{array}{l} LHS: \cot^{2} x - \cot^{2} x \cos^{2} x \\ = \cot^{2} x (1 - \cos^{2} x) \\ = \cot^{2} x (s i n^{2} x) \\ = \frac{\cos^{2} x}{s i n^{2} x} (s i n^{2} x) \\ = \cos^{2} x (RHS) \end{array}

(b)

\begin{array}{l} LHS: \frac{\sec x}{\sec x - \cos x} \\ = \frac{\frac{1}{\cos x}}{\frac{1}{\cos x} - \cos x} = \frac{\frac{1}{\cos x}}{\frac{1}{\cos x} - \frac{\cos^{2} x}{\cos x}} \\ = \frac{\frac{1}{\cos x}}{\frac{1 - \cos^{2} x}{\cos x}} = \frac{1}{\cos x} \times \frac{\cos x}{1 - \cos^{2} x} \\ = \frac{1}{1 - \cos^{2} x} = \frac{1}{s i n^{2} x} \\ = \cos e c^{2} x (RHS) \end{array}

5.1 Positive and Negative Angles

Posted on April 22, 2020 by user

5.1 Positive and Negative Angles

1. Positive angles are angles measure in an anticlockwise rotate from the positive x-axis about the origin, O.

2. Negative angles are angles measured in a clockwise rotation from the positive x-axis about the origin O.

3. One complete revolution is 360° or 2π radians.

Example:

Show each of the following angles on a separate diagram and state the quadrant in which the angle is situated.

(a) 410°

(b) 890°

(c) \frac{22}{9} π radians

(d) \frac{10}{3} π radians

(e) –60^o

(f) –500°

(g) - 3 \frac{1}{4} π radians

Solution:

(a)

410° = 360° + 50°

Based on the above circular diagram, the positive angle of 410° is in the first quadrant.

(b)

890° = 720° + 170°

Based on the above circular diagram, the positive angle of 890° is in the second quadrant.

(c)

\frac{22}{9} π rad = (2 π + \frac{4}{9} π) rad = 360^{o} + 80^{o}

Based on the above circular diagram, the positive angle of

\frac{22}{9} π radians

is in the first quadrant.

(d)

\frac{10}{3} π rad = (3 π + \frac{1}{3} π) rad = 540^{o} + 60^{o}

Based on the above circular diagram, the positive angle of

\frac{10}{3} π radians

is in the third quadrant.

(e)

Based on the above circular diagram, the negative angle of –60° is in the fourth quadrant.

(f)

–500° = –360° – 140°

Based on the above circular diagram, the negative angle of –500° is in the third quadrant.

(g)

- 3 \frac{1}{4} π rad = (- 3 π - \frac{1}{4} π) rad = - 540^{o} - 45^{o}

Based on the above circular diagram, the negative angle of

- 3 \frac{1}{4} π radians

is in the second quadrant.

Short Question 4 – 6

Posted on April 22, 2020 by user

Question 4:
Solve the equation 3 sin A cos A – cos A = 0 for 0° ≤ A ≤ 360°.

Solution:
3 sin A cos A – cos A = 0
cos A (3 sin A – 1) = 0
cos A = 0 or sin A = ⅓

cos A = 0
A = 90°, 270°

sin A = ⅓
Basic angle = 19°28'
A = 19°28', 180° – 19°28'
A = 19°28', 160°32'

Hence A = 19°28', 90°, 160°32', 270°.

Question 5:

Solve the equation 4 sin (x – π) cos (x – π) = 1 for 0^o ≤ x ≤ 360^o.

Solution:

4 sin (x – π) cos (x – π) = 1

2 [2 sin (x – π) cos (x – π)] = 1

2 sin (x – π) cos (x – π) = ½

sin 2(x – π) = ½ ← (sin 2x= 2 sinx cosx)

sin 2(x – 180^o) = ½ ← (π rad = 180^o)

sin (2x – 360^o) = ½

sin 2x cos 360^o – cos 2x sin 360^o = ½

sin 2x (1) – cos 2x (0) = ½ ← (cos 360^o = 1, sin 360^o = 0)

sin 2x = ½

basic angle = 30^o ← (special angle, sin 30^o= ½)

2x = 30^o, 150^o, 390^o, 510^o

x = 15^o, 75^o, 195^o, 255^o

5.3.4 Sketching Graphs of Trigonometric Functions (Part 3)

Posted on April 22, 2020 by user

5.3.4 Sketching Graphs of Trigonometric Functions (Part 3)

Example 2:

(a) Sketch the graph y = –½ cos x for 0 ≤ x ≤ 2π.

(b) Hence, using the same axes, sketch a suitable graph to find the number of solutions to the equation

\frac{π}{2 x} + \cos x = 0

for 0 ≤ x ≤ 2π.

State the number of solutions.

Solution:

(a)

(b)

\begin{array}{l} \frac{π}{2 x} + \cos x = 0 \\ \frac{π}{2 x} = - \cos x \\ \frac{π}{4 x} = - \frac{1}{2} \cos x \leftarrow Multiply both sides by \frac{1}{2} \\ y = \frac{π}{4 x} \leftarrow y = - \frac{1}{2} \cos x \end{array}

The suitable graph to draw is

y = \frac{π}{4 x} .

x	$\frac{π}{2}$	π	2π
$y = \frac{π}{4 x}$	½	¼	⅛

From the graphs, there are two points of intersection for 0 ≤ x ≤ 2π.

Number of solutions = 2.

5.3.2 Sketching Graphs of Trigonometric Functions (Part 1)

Posted on April 22, 2020 by user

5.3.2 Sketching Graphs of Trigonometric Functions

Example 1:

Sketch the graph of each of the following trigonometric functions for π ≤ x ≤ 2π.

(a) y = 3 sin x
(b) y = 2 cos x

Solution:
(a)

(b)

(c)

(d)

(e)

(f)

Basic Trigonometric Identities

Posted on April 22, 2020 by user

5.4 Basic Trigonometric Identities

Three basic trigonometric identities are:

sin² x + cos² x = 1

tan² x + 1 = sec² x

cot² x + 1 = cosec² x

[adinserter block="3"]

Example 1 (To Prove Trigonometric Identities which involve the Three Basic Identities)

Prove each of the following trigonometric identities.

(a) sin²x – cos² x = 1 – 2 cos² x

(b) (1 – cosec²x) (1– sec² x) = 1

Solution:

(a)

sin²x– cos² x = 1 – 2 cos²x

LHS: sin²x – cos² x

= 1 – cos² x – cos² x

= 1 – 2 cos² x (RHS)

(b)

\begin{array}{l} (1 - {cosec}^{2} x) (1 - \sec^{2} x) = 1 \\ LHS: (1 - {cosec}^{2} x) (1 - \sec^{2} x) \\ = (- \cot^{2} x) (- \tan^{2} x) \\ = (\cot^{2} x) (\tan^{2} x) \\ = (\frac{1}{\tan^{2} x}) \tan^{2} x \\ = 1 (RHS) \end{array}

Example 2 (To Solve Trigonometric Equations which involve the Three Basic Identities)

Solve the following trigonometric equations for 0^o≤ x ≤ 360^o.

(a) sin²x cos x + 1 = cos x

(b) 2 cosec²x – 5 cot x = 0

Solution:

(a)

sin²x cos x + 1 = cos x

(1 – cos²x) cos x + 1 = cos x

cos x – cos³x + 1 = cos x

cos³x = 1

cos x = 1

x = 0^o, 360^o

(b)

2 cosec²x – 5 cot x = 0

2 (1 + cot²x) – 5 cot x = 0

2 + 2 cot²x – 5 cot x = 0

2 cot²x – 5 cot x + 2 = 0

(2 cotx – 1) (cot x – 2) = 0

cotx= ½ or cotx = 2

tan x = 2 tan x = ½

x =63.43^o, 243.43^o x = 26.57^o, 206.57^o

(Note: tangent is positive in the first and third quadrants)

Thus, x = 26.57^o, 63.43^o, 206.57^o, 243.43^o

5.3.1 General Pattern for y = sinx, y = cosx and y = tanx

Posted on April 22, 2020 by user

5.3.1 General pattern for y = sin x , y = cos x and y = tan x

(1) Graph of y = sin x

x	0^o	90^o	180^o	270^o	360^o
sin x	0	1	0	-1	0

(2) Graph of y = cos x

x	0^o	90^o	180^o	270^o	360^o
cos x	1	0	-1	0	1

(3) Graph of y = tan x

x	0^o	90^o	180^o	270^o	360^o
tan x	0	¥	0	¥	0

Short Question 6 & 7

Posted on April 22, 2020 by user

Question 6:
The points P, Q and R are collinear. It is given that $\vec{P Q} = 4 \underset{˜}{a} - 2 \underset{˜}{b}$ and

\vec{Q R} = 3 \underset{˜}{a} + (1 + k) \underset{˜}{b}

, where k is a constant. Find
(a) the value of k,
(b) the ratio of PQ : QR.

Solution:
(a)

\begin{array}{l} Note: If P, Q and R are collinear, \\ \vec{P Q} = m \vec{Q R} \\ 4 \underset{˜}{a} - 2 \underset{˜}{b} = m [3 \underset{˜}{a} + (1 + k) \underset{˜}{b}] \\ 4 \underset{˜}{a} - 2 \underset{˜}{b} = 3 m \underset{˜}{a} + m (1 + k) \underset{˜}{b} \\ Comparing vector: \\ \underset{˜}{a} : 4 = 3 m \\ m = \frac{4}{3} \\ \underset{˜}{b} : - 2 = m (1 + k) \\ - 2 = \frac{4}{3} (1 + k) \\ 1 + k = - \frac{6}{4} \\ k = - \frac{3}{2} - 1 \\ k = - \frac{5}{2} \end{array}

(b)

\begin{array}{l} \vec{P Q} = m \vec{Q R} \\ \vec{P Q} = \frac{4}{3} \vec{Q R} \\ \frac{\vec{P Q}}{\vec{Q R}} = \frac{4}{3} \\ ∴ P Q : Q R = 4 : 3 \end{array}

Question 7:
Given that

\underset{˜}{x} = 3 \underset{˜}{i} + m \underset{˜}{j}

and

\underset{˜}{y} = 4 \underset{˜}{i} - 3 \underset{˜}{j}

, find the values of m if the vector

\underset{˜}{x}

is parallel to the vector

\underset{˜}{y}

.

Solution:

\begin{array}{l} If vector \underset{˜}{x} is parallel to vector \underset{˜}{y} \\ \underset{˜}{x} = h \underset{˜}{y} \\ (3 \underset{˜}{i} + m \underset{˜}{j}) = h (4 \underset{˜}{i} - 3 \underset{˜}{j}) \\ 3 \underset{˜}{i} + m \underset{˜}{j} = 4 h \underset{˜}{i} - 3 h \underset{˜}{j} \\ Comparing vector: \\ \underset{˜}{i} : 3 = 4 h \\ h = \frac{3}{4} \\ \underset{˜}{j} : m = - 3 h \\ m = - 3 (\frac{3}{4}) = - \frac{9}{4} \end{array}

4.2 Multiplication of Vector by a Scalar and the Parallel Condition of Two Vectors

Posted on April 22, 2020 by user

4.2 Multiplication of Vector by a Scalar and the Parallel Condition of Two Vectors

1. When a vector

\underset{˜}{a}

is multiplied by a scalar k, the product is

k \underset{˜}{a}

. Its magnitude is k times the magnitude of the vector

\underset{˜}{a}

2. The vector

\underset{˜}{a}

is parallel to the vector

\underset{˜}{b}

if and only if

\underset{˜}{b} = k \underset{˜}{a}

, where k is a constant.

3. If the vectors

\underset{˜}{a}

and

\underset{˜}{b}

are not parallel and

h \underset{˜}{a} = k \underset{˜}{b}

, then h = 0 and k = 0.

Example 1:

If vectors

\underset{˜}{a} and \underset{˜}{b}

are not parallel and

(k - 7) \underset{˜}{a} = (5 + h) \underset{˜}{b}

, find the value of k and of h.

Solution:

The vectors

\underset{˜}{a} and \underset{˜}{b}

are not parallel, so

k – 7 = 0 → k = 7

5 + h = 0 → h = –5