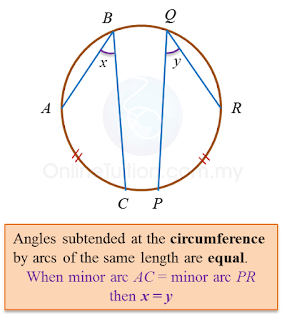
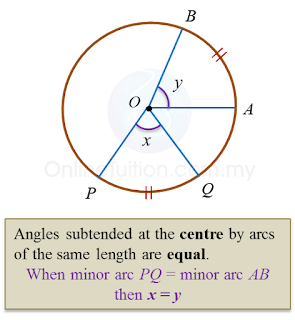
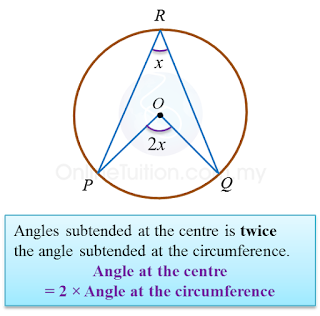
3.2.2 Circles II, PT3 Practice
Question 6:
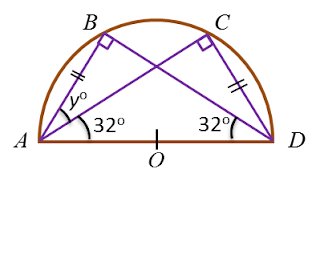
Diagram below shows a circle with centre O. POR is a straight line.
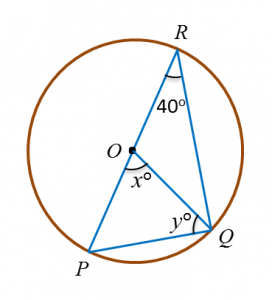

Find the value of x and of y.
Solution:
x=40×2=80y=180−802=50
[adinserter block="5"]
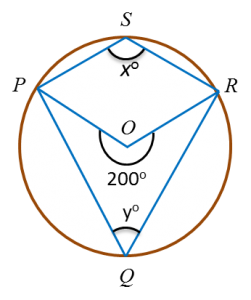
Question 7:
Diagram below shows a circle with centre O.
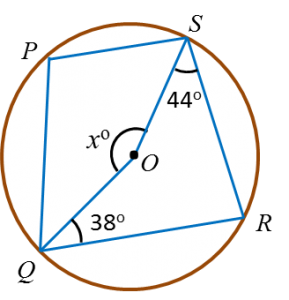
Find the value of x.
Solution:
∠QRO=38o,∠ORS=44o∠QRS=38o+44o=82oxo=82o×2xo=164ox=164
Diagram below shows a circle with centre O.

Find the value of x.
Solution:
∠QRO=38o,∠ORS=44o∠QRS=38o+44o=82oxo=82o×2xo=164ox=164
[adinserter block="5"]
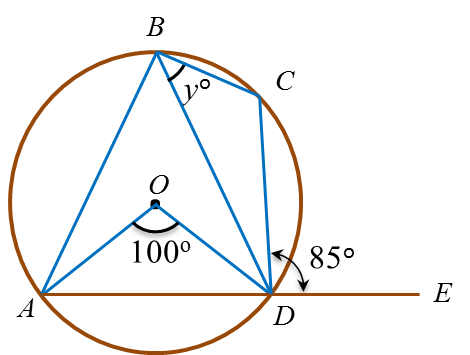
Question 8:
Diagram below shows a circle. QTS is the diameter of the circle and PTR is a straight line.
 Find the value of x.
Find the value of x.
Solution:
∠RPS=90o−42o=48oxo=48o+37o=85o
Diagram below shows a circle. QTS is the diameter of the circle and PTR is a straight line.
 Find the value of x.
Find the value of x.Solution:
∠RPS=90o−42o=48oxo=48o+37o=85o
[adinserter block="5"]
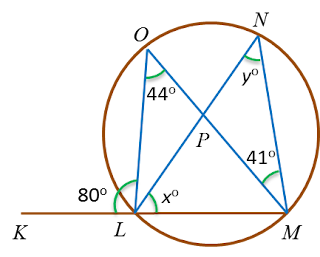
Question 9:
Diagram below shows a circle with centre O. PQR is a straight line.
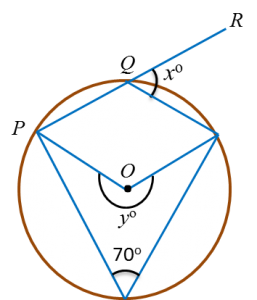
Find the value of x and of y.
Solution:
In cyclic quadrilateral,
Angle at Q = 180o – 70o = 110o
x2 = exterior angle at Q
= 180o – 110o
= 70o
x = 70
yo = reflex angle at O
yo = 360o – (70o × 2)
yo = 220o
y = 220
Diagram below shows a circle with centre O. PQR is a straight line.

Find the value of x and of y.
Solution:
In cyclic quadrilateral,
Angle at Q = 180o – 70o = 110o
x2 = exterior angle at Q
= 180o – 110o
= 70o
x = 70
yo = reflex angle at O
yo = 360o – (70o × 2)
yo = 220o
y = 220
Question 10:
In diagram below, ABCD and DEFG are straight lines.
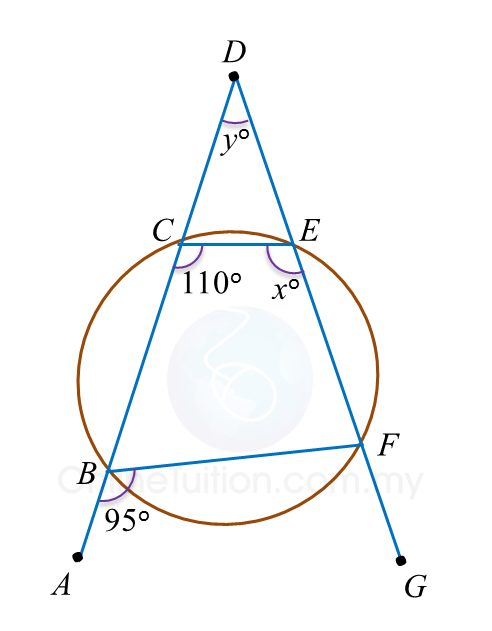 Find the value of x and of y.
Find the value of x and of y.
Solution:
x=95(exterior of ∠B cyclic quadrilateral)∠CBF+∠BFE+95o+110o=360o ∠CBF+∠BFE+205o=360o∠CBF+∠BFE=155oyo+∠CBF+∠BFE=180o yo+155o=180o y=25
In diagram below, ABCD and DEFG are straight lines.
 Find the value of x and of y.
Find the value of x and of y.Solution:
x=95(exterior of ∠B cyclic quadrilateral)∠CBF+∠BFE+95o+110o=360o ∠CBF+∠BFE+205o=360o∠CBF+∠BFE=155oyo+∠CBF+∠BFE=180o yo+155o=180o y=25