Question 6:
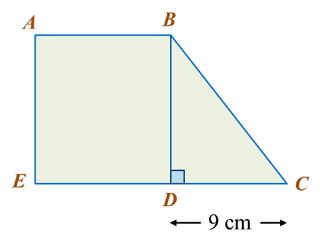
In diagram below, ABCH is a square and DEFG is a rectangle. HCDE is a straight line and HC = CD.

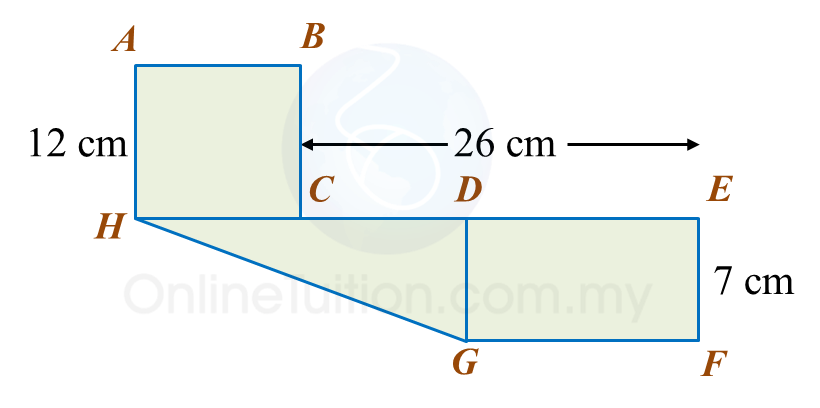
Find the perimeter, in cm, of the whole diagram.

Solution:
$\begin{array}{l}G{H}^2=D{H}^2+D{G}^2\\ ={24}^2+7^2\\ =576+49\\ =625\\ GH=25\text{ cm}\\ \\ \text{Perimeter of the whole diagram}\\ =12+12+12+26+7+14+25\\ =108\text{ cm}\end{array}$

Question 7:
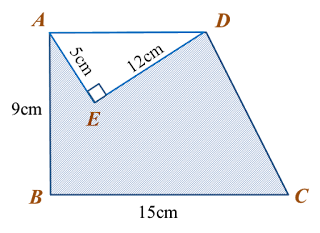
In the diagram, ABC and EFD are right-angled triangles.


Calculate the perimeter, in cm, of the shaded region.

Solution:
$\begin{array}{l}D{E}^2=3^2+4^2\\ =9+16\\ =25\\ DE=\sqrt{25}\\ =5\text{ cm}\\ \\ A{C}^2=7^2+{24}^2\\ =49+576\\ =625\\ AC=\sqrt{625}\\ =25\text{ cm}\\ \\ \text{Perimeter of the shaded region}\\ =24+7+\left(25-5\right)+3+4\\ =58\text{ cm}\end{array}$

Question 8:
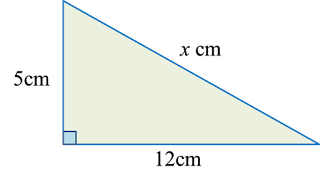
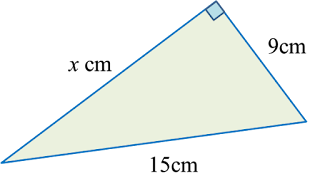
In the diagram, ABD and BCE are right-angled triangles. ABC and DBE are straight lines. The length of AB is twice the length of BC.


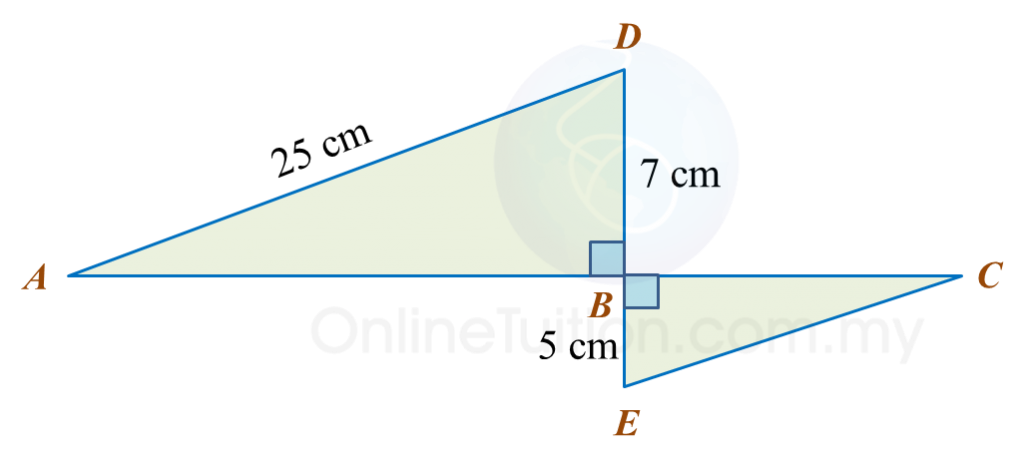
Calculate the length, in cm, of CE.

Solution:
$\begin{array}{l}A{B}^2={25}^2-7^2\\ =625-49\\ =576\\ AB=\sqrt{576}\\ =24\text{ cm}\\ BC=24\text{ cm}÷2\\ =12\text{ cm}\\ C{E}^2=5^2+{12}^2\\ =25+144\\ =169\\ CE=\sqrt{169}\\ =13\text{ cm}\end{array}$

Question 9:
Diagram below shows two right-angled triangles, ABE and CBD. BED is a straight line.
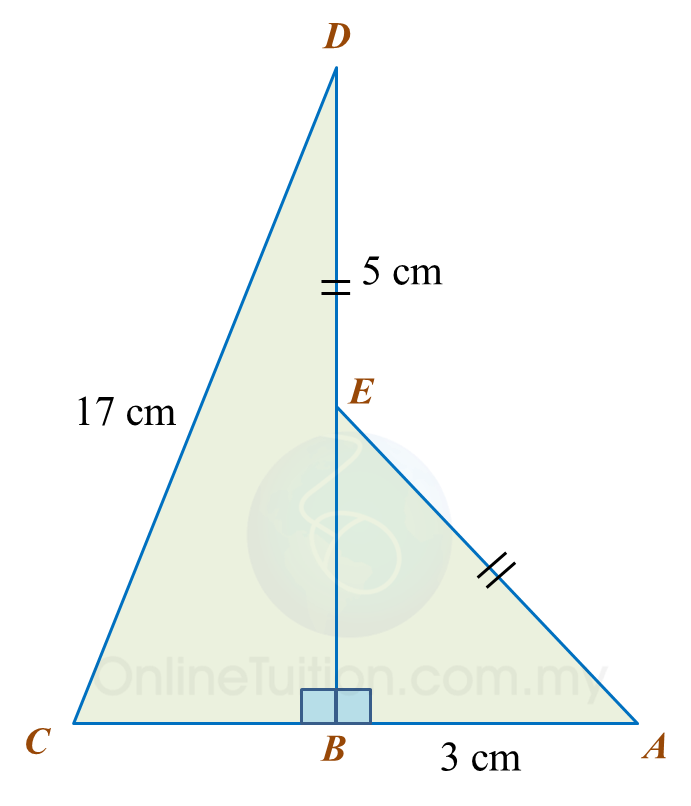Find the length, in cm, of BC. Round off the answer to two decimal places.

Solution:
$\begin{array}{l}{3}^2+B{E}^2=5^2\\ B{E}^2=5^2-3^2=16\\ BE=4\text{ cm}\\ \\ B{C}^2+{\left(5+4\right)}^2={17}^2\\ B{C}^2={17}^2-9^2=208\\ BC=\sqrt{208}\\ BC=14.42\text{ cm}\end{array}$

Question 10:
Diagram below shows two right-angled triangles, ABC and ADE. ACD is a straight line.
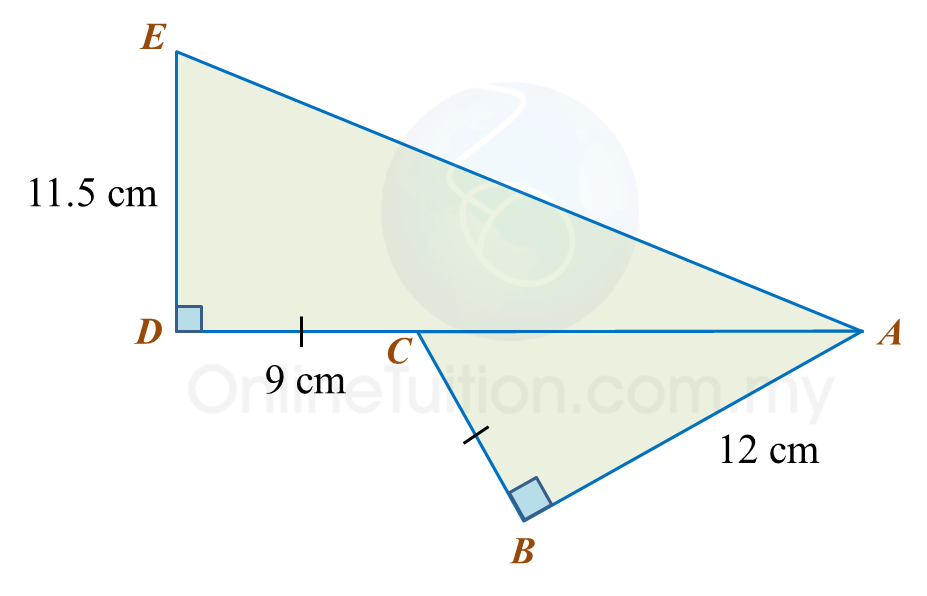Find the length, in cm, of AE. Round off the answer to one decimal places.

Solution:
$\begin{array}{l}A{C}^2={12}^2+9^2\\ =225\\ AC=\sqrt{225}\\ =15\text{ cm}\\ \\ A{E}^2={\left(15+9\right)}^2+{11.5}^2\\ =576+132.25\\ =708.25\\ AE=\sqrt{708.25}\\ =26.6\text{ cm}\end{array}$

In a triangle, if the sum of the squares of the two sides   is equal to the square of the longest side, then the angle   opposite the longest side is a right angle.