12.2.2 Solid Geometry (II), PT3 Focus Practice

Question 5:
Sphere below has a surface area of 221.76 cm².


Calculate its radius.
$\left(\pi =\frac{22}{7}\right)$

Solution:
Surface area of sphere = 4πr²
$\begin{array}{l}4\pi r^2=221.76\\ 4\times \frac{22}{7}\times r^2=221.76\\ r^2=\frac{221.76\times 7}{4\times 22}\\ r^2=17.64\\ r=\sqrt{17.64}\\ r=4.2\text{cm}\end{array}$

Question 6:
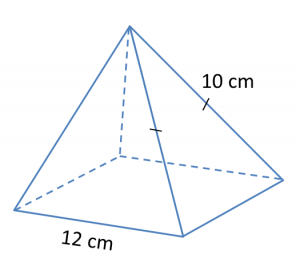
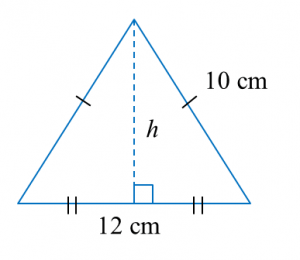
Diagram below shows a right pyramid with a square base.

Given the height of the pyramid is 4 cm.
Calculate the total surface area, in cm², of the right pyramid.

Solution:
h² = 3² + 4²
= 9 + 16
= 25
h = √25   = 5 cm²

Total surface area of the right pyramid
= Base area + 4 (Area of triangle)
= (6 × 6) + 4 × 4 (½ × 6 × 5)
= 36 + 60
= 96 cm²

Question 7:
Diagram below shows a prism.

Draw to full scale, the net of the prism on the grid in the answer space. The grid has equal squares with sides of 1 unit.

Solution:

$\begin{array}{l}=\left(2\times \frac{22}{7}\times 7^2\right)+\left(2\times \frac{22}{7}\times 7\times 20\right)\\ =308+880\\ =1188c{m}^2\end{array}$

= 132 cm²

Question 4:
$\begin{array}{l}=\frac{22}{7}\times 3.5\times 6\\ =66c{m}^2\end{array}$