2.1 Squares, Square Roots, Cube and Cube Roots
(A) Squares
The square of a number is the answer you get when you multiply a number by itself.
Example:
(e)(312)2=(72)2=72×72=494(f)(−127)2=(−97)2=(−97)×(−97)=8149
(B) Perfect Squares
1. Perfect squares are the squares of whole numbers.
2. Perfect squares are formed by multiplying a whole number by itself.
Example:
4 = 2 × 2 9 = 3 × 3 16 = 4 × 4
3. The first twelve perfect squares are:
= 12, 22, 32, 42, 52, 62, 72, 82, 92, 102, 112, 122
= 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144
(C) Square Roots
1. The square root of a positive number is a number multiplied by itself whose product is equal to the given number.
Example:
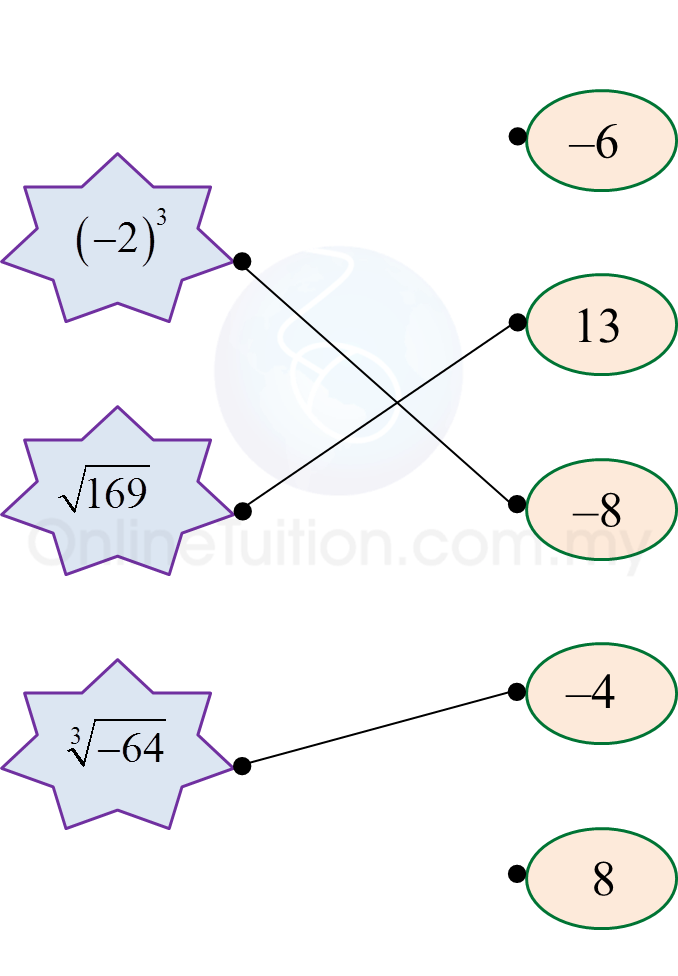
(a)√169=√13×13=13(b)√2564=√5×58×8=58(c)√7298=√72369849=√6×67×7=67(d)√3116=√4916=74=134(e)√1.44=√1441110025=√3625=65=115
(D) Cubes
1. The cube of a number is obtained when that number is multiplied by itself twice.
Example:
The cube of 3 is written as
33 = 3 × 3 × 3
= 27
2. The cube of a negative number is negative.
Example:
(–2)3 = (–2) × (–2) × (–2)
= –8
3. The cube of zero is zero. The cube of one is one, 13 = 1.
(E) Cube Roots
1. The cube root of a number is a number which, when multiplied by itself twice, produces the particular number.
is the symbol for cube root.
Example:
is read as ‘cube root of sixty-four’.
2. The cube root of a positive number is positive.
Example:
3. The cube root of a negative number is negative.
Example:
4. To determine the cube roots of fractions, the fractions should be simplified to numerators and denominators that are cubes of integers.
Example: