Question 10:
A technician needs to climb a ladder to repair a street lamp as shown in Diagram below.
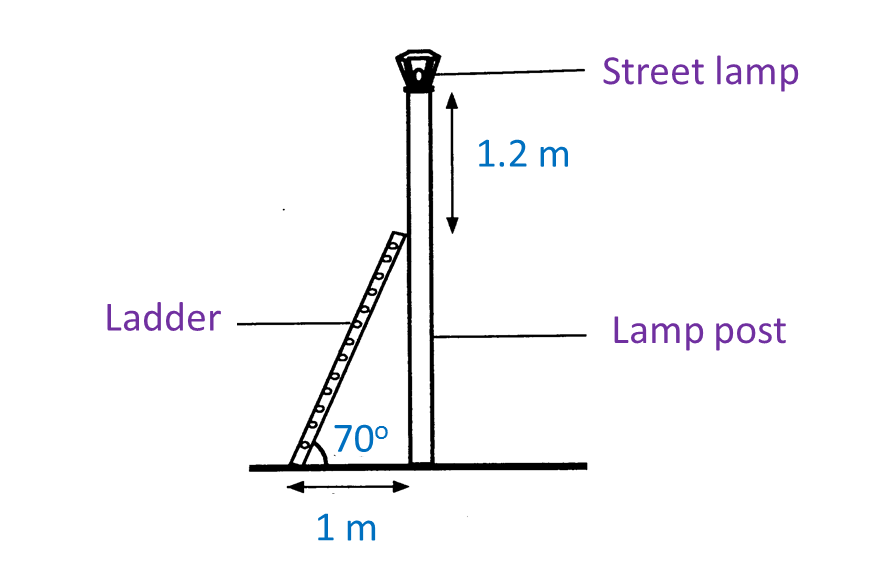 (a) What is the length, in m, of the ladder?
(a) What is the length, in m, of the ladder?
(b) Find the height, in m, of the lamp post.
Solution:
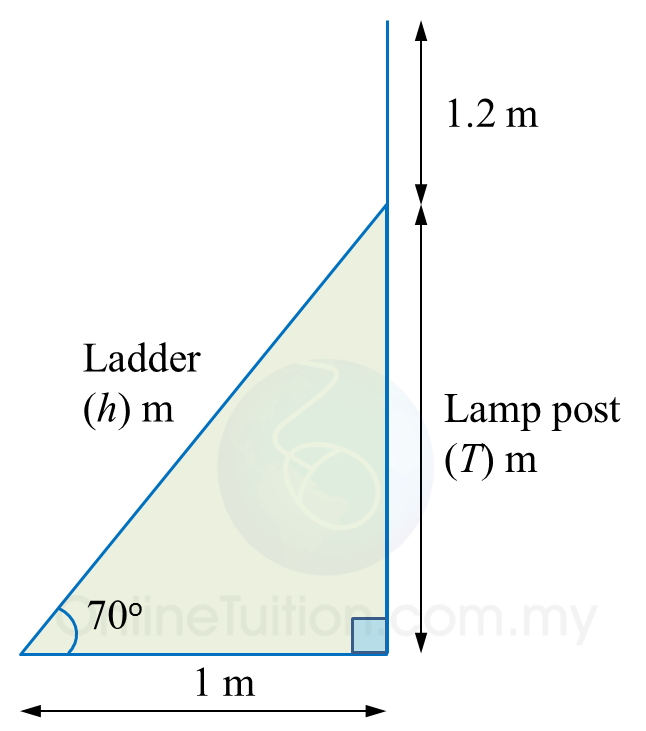
(a)
cos70o=1hh=1cos70oh=10.342h=2.924 mHence, the length of the ladder=2.924 m
(b)
tan70o=T1T=tan70o×1 =2.747×1 =2.747 mThe height of the lamp post=2.747+1.2=3.947 m
A technician needs to climb a ladder to repair a street lamp as shown in Diagram below.
 (a) What is the length, in m, of the ladder?
(a) What is the length, in m, of the ladder?(b) Find the height, in m, of the lamp post.
Solution:

(a)
cos70o=1hh=1cos70oh=10.342h=2.924 mHence, the length of the ladder=2.924 m
(b)
tan70o=T1T=tan70o×1 =2.747×1 =2.747 mThe height of the lamp post=2.747+1.2=3.947 m


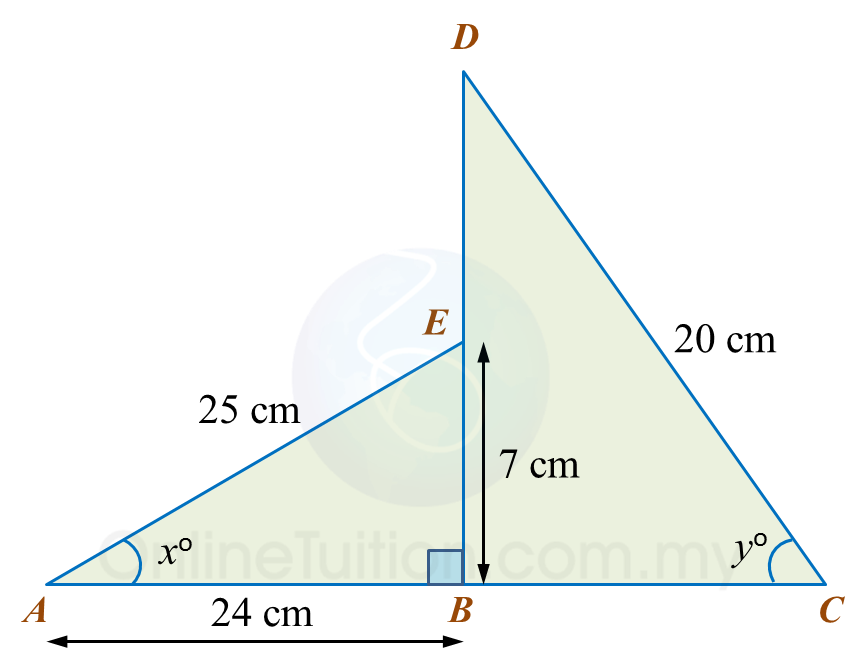
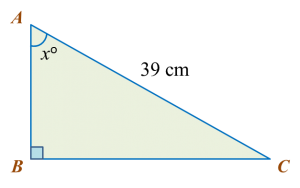
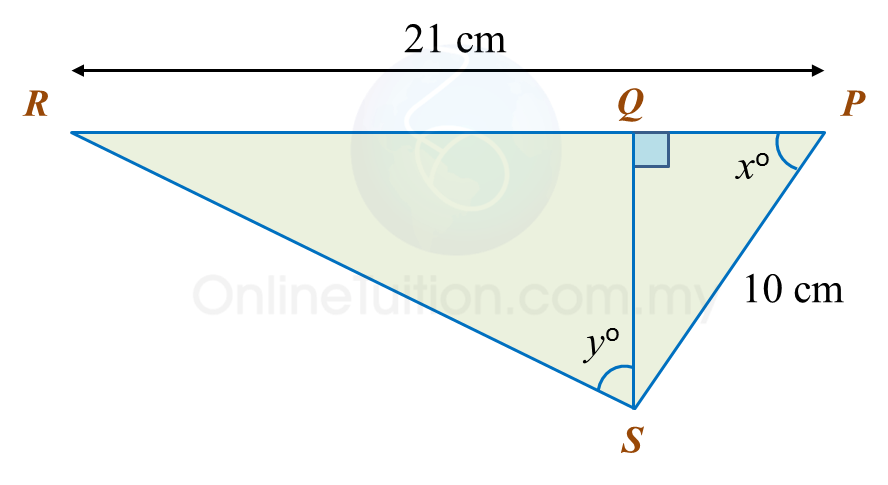
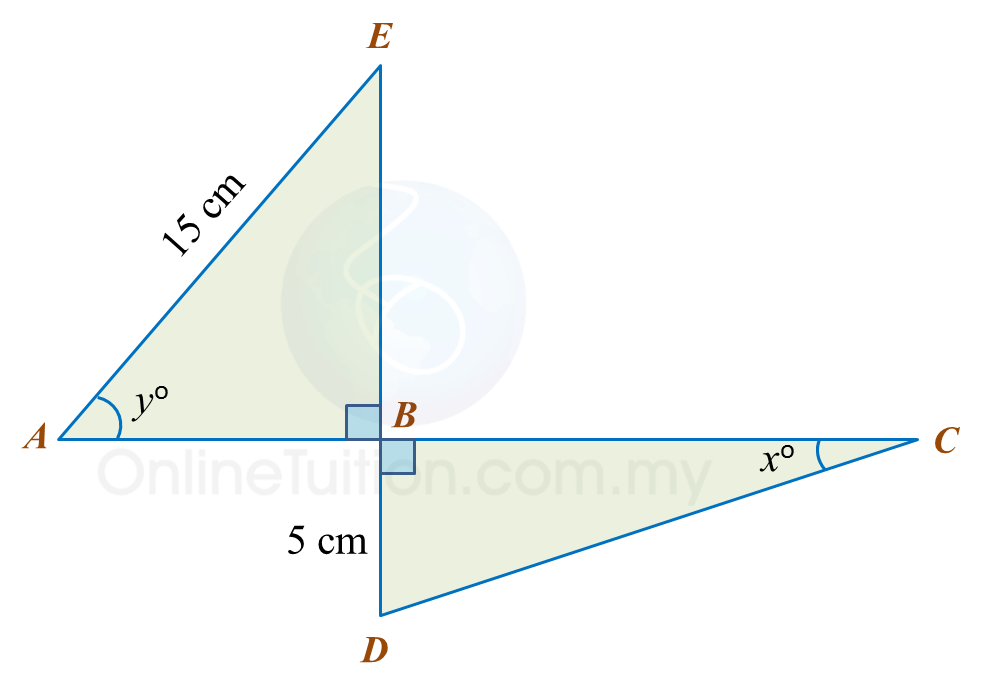
 Calculate
Calculate