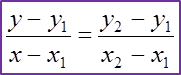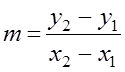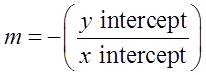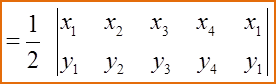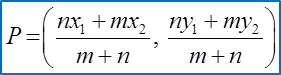Question 1:
Solution:
(a)
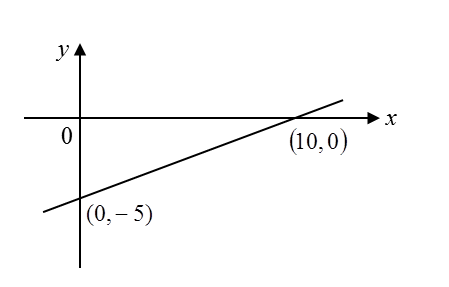
Equation of PQ2y−x−5=02y=x+5y=12x+52∴mPQ=12In a trapizium, mPQ=mSR12=0−(−3)w−4w−4=6w=10
(b)
mPQ=12mPS=−1mPQ=−112=−2
(c)
Let M=(x,y)Given that △QMS is perpendicular at MThus △QMS=90∘(mQM)(mMS)=−1(y−5x−5)(y−(−3)x−4)=−1(y−5)(y+3)=−1(x−5)(x−4)y2+3y−5y−15=−1(x2−4x−5x+20)y2−2y−15=−x2+9x−20x2+y2−9x−2y+5=0
Hence, the equation of locus of the moving point M is
The diagram shows a trapezium PQRS. Given the equation of PQ is 2y – x – 5 = 0, find
(a) The value of w,
(b) the equation of PS and hence find the coordinates of P.
(c) The locus of M such that triangle QMS is always perpendicular at M.
Solution:
(a)
Equation of PQ2y−x−5=02y=x+5y=12x+52∴mPQ=12In a trapizium, mPQ=mSR12=0−(−3)w−4w−4=6w=10
(b)
mPQ=12mPS=−1mPQ=−112=−2
Point S = (4, –3), m = –2
y – y1 = m (x– x1)
y – (–3) = –2 (x – 4)
y + 3 = –2x + 8
y = –2x + 5
Equation of PS is y = –2x + 5
PS is y = –2x + 5-----(1)
PQ is 2y = x + 5-----(2)
Substitute (1) into (2)
2 (–2x + 5) = x + 5
–4x + 10 = x + 5
–5x = –5
x = 1
From (1), y = –2(1) + 5
y = 3
Coordinates of point P = (1, 3).
(c)
Let M=(x,y)Given that △QMS is perpendicular at MThus △QMS=90∘(mQM)(mMS)=−1(y−5x−5)(y−(−3)x−4)=−1(y−5)(y+3)=−1(x−5)(x−4)y2+3y−5y−15=−1(x2−4x−5x+20)y2−2y−15=−x2+9x−20x2+y2−9x−2y+5=0
Hence, the equation of locus of the moving point M is
x2 + y2– 9x – 2y + 5 = 0.