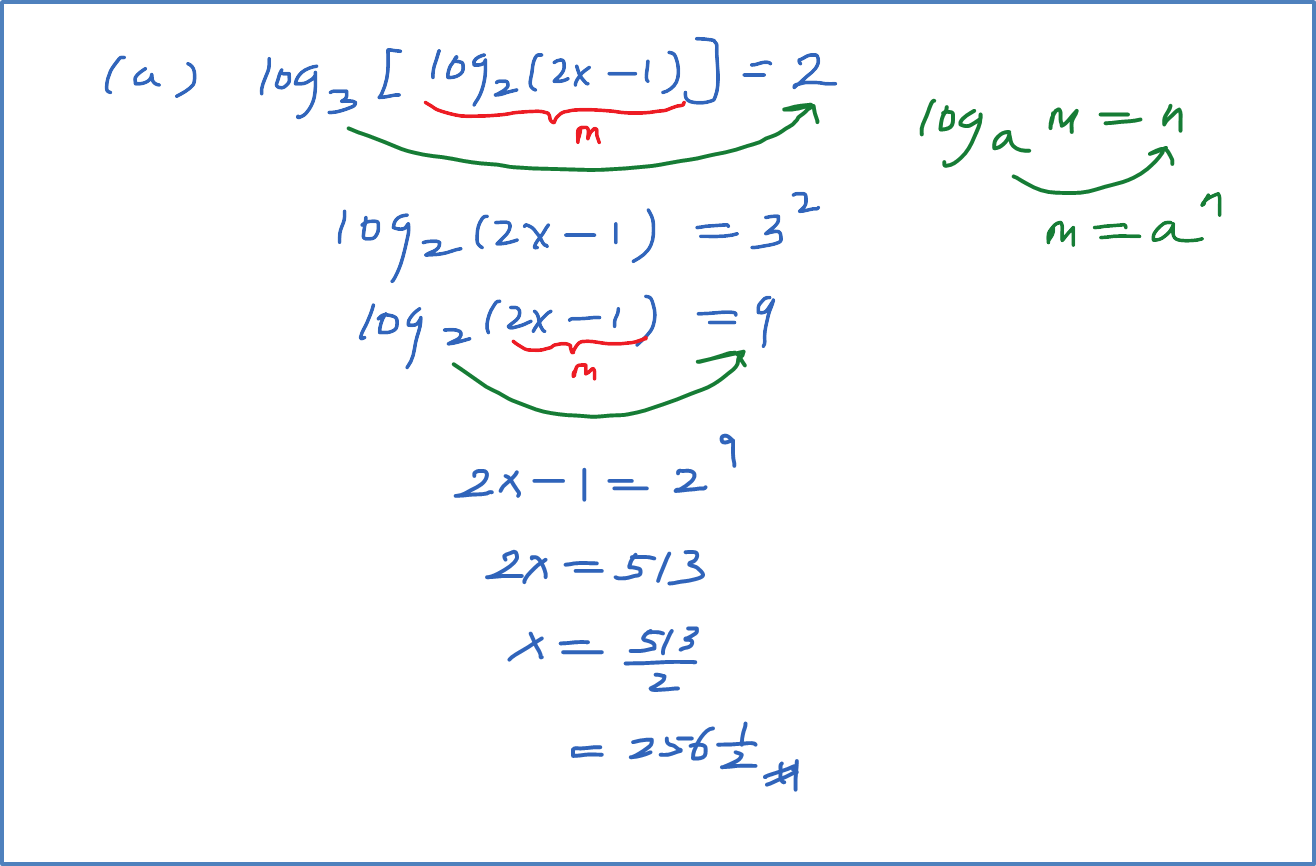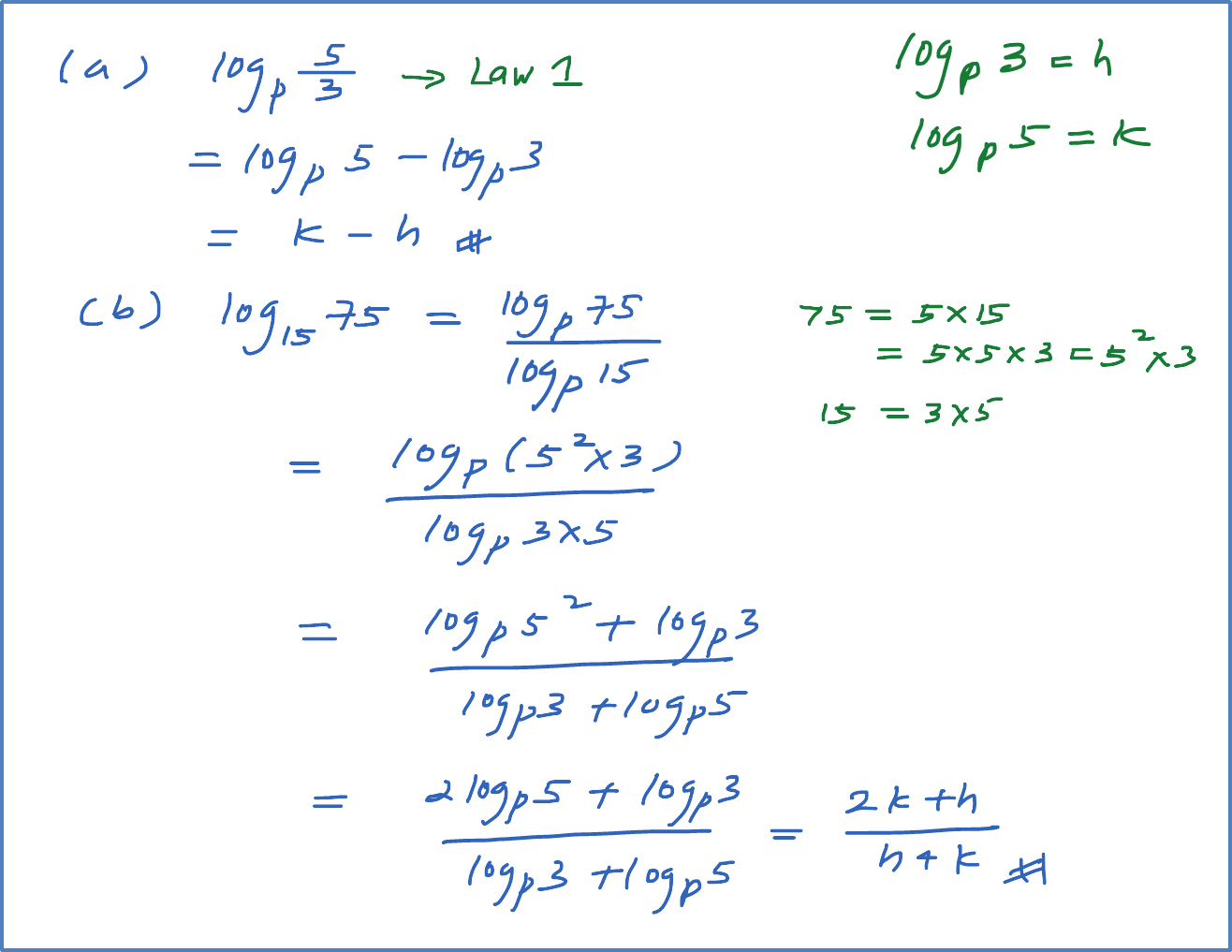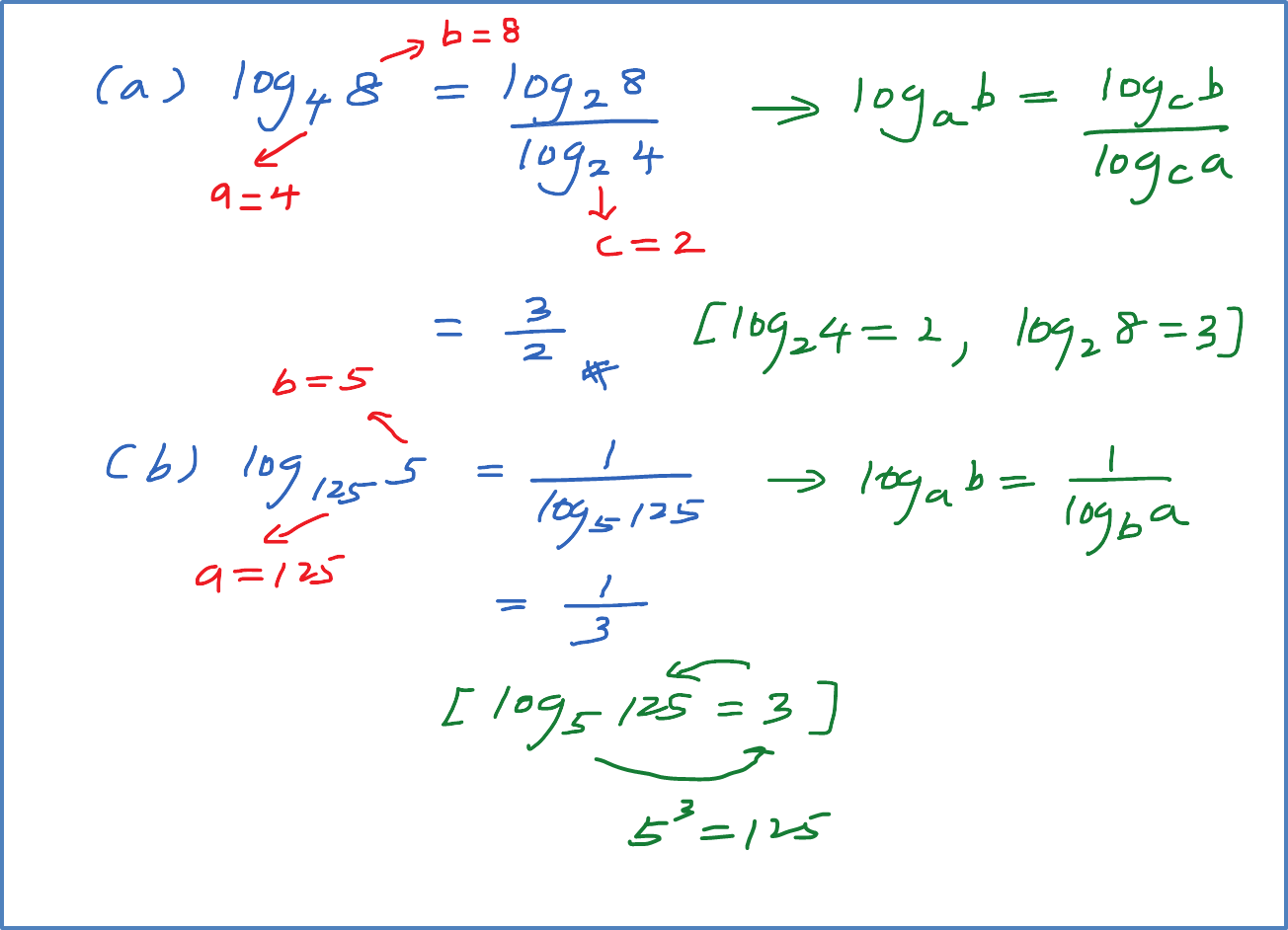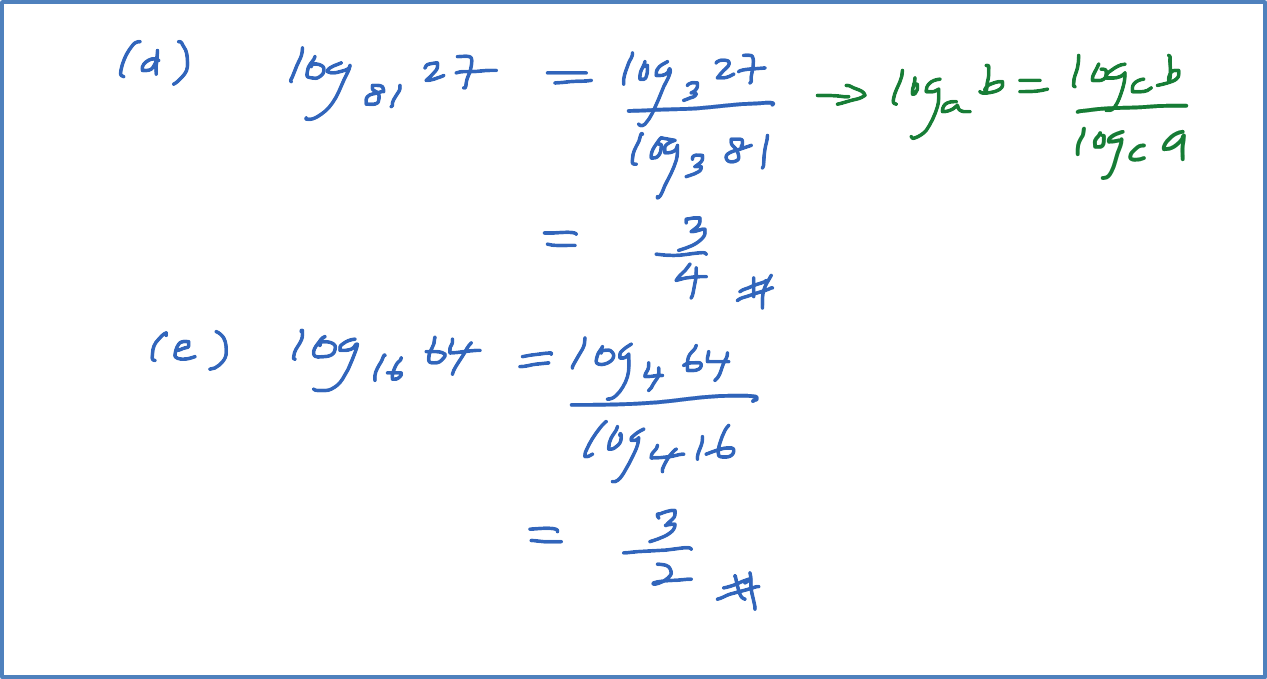5.4 Equations Involving Logarithms (Example 2 & 3)

Posted on April 21, 2020 by user

Example 2
Solve the following equation:
(a)

\log_{\sqrt{y}} 81 - 3 = \log_{\sqrt{y}} 3

(b)

2 \log_{2} x - \log_{2} (\frac{x}{2} - 1) - 4 = 0

Example 3
Sole the following equation:
(a)

\log_{3} [\log_{2} (2 x - 1)] = 2

(b)

\log_{16} [\log_{2} (5 x - 4)] = \log_{9} \sqrt{3}

5.4 Equations Involving Logarithms

Posted on April 21, 2020 by user

METHOD:

For two logarithms of the same base, if $\log_{a} m = \log_{a} n$ , then m = n .
Convert to index form, if $\log_{a} m = n$ , then m = aⁿ.

Example 1
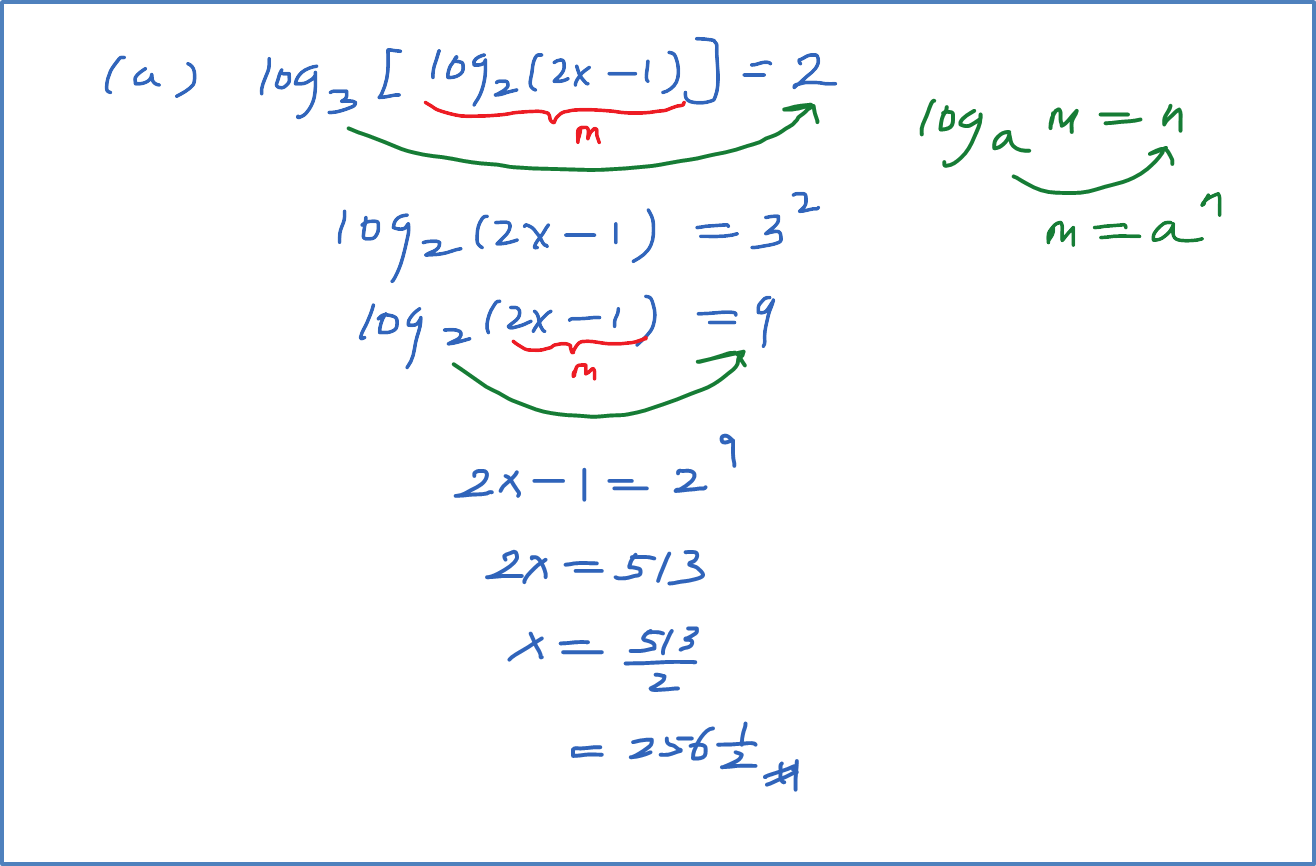
Solve the following equation:
(a)

\log_{3} 2 + \log_{3} (x + 5) = \log_{3} (3 x - 1)

(b)

\log_{2} 8 x - 3 = \log_{2} (2 x - 1)

(c)

3 \log_{x} 2 + 2 \log_{x} 4 - \log_{x} 256 = - 1

5.3 Equations Involving Indices (Example 5)

Posted on April 21, 2020 by user

Example 5 (Unequal Base – put log both sides):
Solve each of the following.
(a)

3^{x + 1} = 7

(b)

2 (3^{x}) = 5

(c)

2^{x} {.3}^{x} = 9^{x - 4}

(d)

5^{x - 1} {.3}^{x + 2} = 10

5.3 Equations Involving Indices (Example 4)

Posted on April 21, 2020 by user

Example 4 (Index Equation - Substitution)
Solve each of the following.
(a)

3^{x - 1} + 3^{x} = 12

(b)

2^{x} + 2^{x + 3} = 72

(c)

4^{x + 1} + 2^{2 x} = 20

5.3 Equations Involving Indices (Example 3)

Posted on April 21, 2020 by user

Example 3 (Index Equation - Equal Base)
Solve each of the following.
(a)

27 (81^{3 x}) = 1

(b)

\sqrt{81^{n + 2}} = \frac{1}{3^{n} 27^{n - 1}}

(c)

8^{x - 1} = \frac{4}{\sqrt{2^{x + 3}}}

5.3 Equations Involving Indices

Posted on April 21, 2020 by user

METHOD:

Comparison of indices or base

If the base are the same , when $a^{x} = a^{y}$ , then x = y
If the index are the same , when $a^{x} = b^{x}$ , then a = b

Using common logarithm (If base and index are NOT the same)

\begin{array}{l} a^{x} = b \Rightarrow \lg a^{x} = \lg b \\ x = \frac{\lg b}{\lg a} \end{array}

Example 1 (Index Equation - Equal base)
Solve each of the following.
(a)

16^{x} = 8

(b)

9^{x} {.3}^{x - 1} = 81

(c)

5^{n + 1} = \frac{1}{125^{n - 1}}

Example 2 (Solving index equation simultaneously)
Solve the following simultaneous equations.

2^{x} {.4}^{2 y} = 8

\frac{3^{x}}{9^{y}} = \frac{1}{27}

5.2b Change of Base of Logarithms (Example 5)

Posted on April 21, 2020 by user

Example 5:

Given that log₂ 3 = 1.585 and log₂ 5 = 2.322, evaluate the following.

log₈ 15
log₅ 0.6
log₁₅ 30
log₁₆ 45

Solution:

5.2b Change of Base of Logarithms (Example 3 & 4)

Posted on April 21, 2020 by user

Example 3
Given that

\log_{p} 3 = h

and

\log_{p} 5 = k

, express the following in term of h and or k.
(a)

\log_{p} \frac{5}{3}

(b)

\log_{15} 75

Example 4
Given that

\log_{3} x = b

, express

\log_{x} 9 x

in term of b.

5.2b Change of Base of Logarithms

Posted on April 21, 2020 by user

Change of Base of Logarithms

\log_{a} b = \frac{\log_{c} b}{\log_{c} a} and \log_{a} b = \frac{1}{\log_{b} a}

Example 1:
Find the value of the following:
a.

\log_{25} 100

b.

\log_{3} 0.45

Answer:

\begin{array}{l} (a) \log_{25} 100 = \frac{\log_{10} 100}{\log_{10} 25} = \frac{\log_{10} 10^{2}}{\log_{10} 25} = \frac{2}{1.3979} = 1.431 \\ (b) \log_{3} 0.45 = \frac{\log_{10} 0.45}{\log_{10} 3} = \frac{- 0.3468}{0.4771} = - 0.727 \end{array}

Example 2
Find the value of the following.
(a)

\log_{4} 8

(b)

\log_{125} 5

(c)

\log_{81} 27

(d)

\log_{16} 64

5.2a Laws of Logarithms (Example 3)

Posted on April 21, 2020 by user

Example 3

Given that

\log_{7} 4 = 0.712

and

\log_{7} 5 = 0.827

, evaluate the following.
(a)

\log_{7} 20

(b)

\log_{7} 1 \frac{1}{4}

(c)

\log_{7} 0.8

(d)

\log_{7} 28

(e)

\log_{7} 140

(f)

\log_{7} 100

(g)

\log_{7} 0.25

(h)

\log_{7} \frac{35}{64}