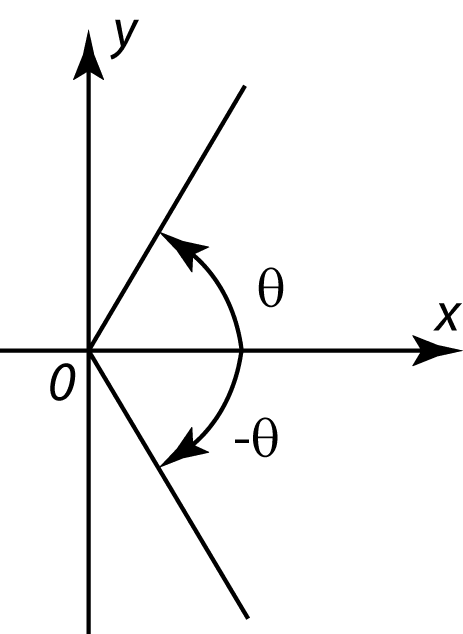
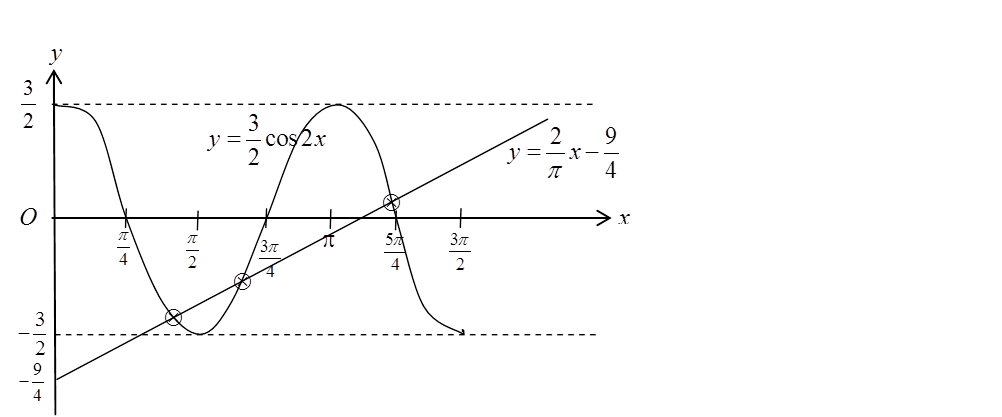
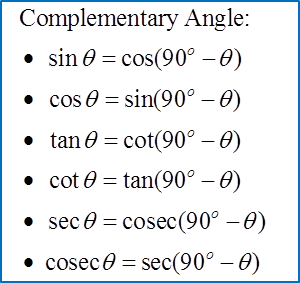
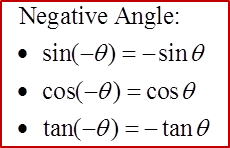5.5.1 Proving Trigonometric Identities Using Addition Formula And Double Angle Formulae (Part 1)

Posted on April 22, 2020 by user

Example 2:

Prove each of the following trigonometric identities.

$\begin{array}{l} (a) \frac{1 + \cos 2 x}{\sin 2 x} = \cot x \\ (b) \cot A \sec 2 A = \cot A + \tan 2 A \\ (c) \frac{s i n x}{1 - c o s x} = \cot \frac{x}{2} \end{array}$

Solution:

(a)

$\begin{array}{l} L H S \\ = \frac{1 + \cos 2 x}{\sin 2 x} \\ = \frac{1 + (2 \cos^{2} x - 1)}{2 \sin x \cos x} \\ = \frac{2 \cos^{2} x}{2 \sin x \cos x} \\ = \frac{\cos x}{\sin x} \\ = \cot x = R H S (proven) \end{array}$

(b)

$\begin{array}{l} R H S \\ = \cot A + \tan 2 A \\ = \frac{\cos A}{\sin A} + \frac{\sin 2 A}{\cos 2 A} \\ = \frac{\cos A \cos 2 A + \sin A \sin 2 A}{\sin A \cos 2 A} \\ = \frac{\cos A (\cos^{2} A - \sin^{2} A) + \sin A (2 \sin A \cos A)}{\sin A \cos 2 A} \\ = \frac{\cos^{3} A - \cos A \sin^{2} A + 2 \sin^{2} A \cos A}{\sin A \cos 2 A} \\ = \frac{\cos^{3} A + \cos A \sin^{2} A}{\sin A \cos 2 A} \\ = \frac{\cos A (\cos^{2} A + \sin^{2} A)}{\sin A \cos 2 A} \\ = \frac{\cos A}{\sin A \cos 2 A} \leftarrow \sin^{2} A + \cos^{2} A = 1 \\ = (\frac{\cos A}{\sin A}) (\frac{1}{\cos 2 A}) \\ = \cot A \sec 2 A \end{array}$

(c)

$\begin{array}{l} L H S \\ = \frac{s i n x}{1 - c o s x} \\ = \frac{2 s i n \frac{x}{2} \cos \frac{x}{2}}{1 - (1 - 2 s i n^{2} \frac{x}{2})} \leftarrow \begin{array}{l} \sin x = 2 s i n \frac{x}{2} \cos \frac{x}{2}, \\ \cos x = 1 - 2 \sin^{2} \frac{x}{2} \end{array} \\ = \frac{2 s i n \frac{x}{2} \cos \frac{x}{2}}{2 s i n^{2} \frac{x}{2}} = \frac{\cos \frac{x}{2}}{s i n \frac{x}{2}} \\ = \cot \frac{x}{2} = R H S (proven) \end{array}$

5.1 Positive and Negative Angles

Posted on April 22, 2020 by user

5.1 Positive and Negative Angles

1. Positive angles are angles measure in an anticlockwise rotate from the positive x-axis about the origin, O.

2. Negative angles are angles measured in a clockwise rotation from the positive x-axis about the origin O.

3. One complete revolution is 360° or 2π radians.

Example:

Show each of the following angles on a separate diagram and state the quadrant in which the angle is situated.

(a) 410°

(b) 890°

$(c) \frac{22}{9} π radians$

$(d) \frac{10}{3} π radians$

(e) –60^o

(f) –500°

$(g) - 3 \frac{1}{4} π radians$

Solution:

(a)

410° = 360° + 50°

Based on the above circular diagram, the positive angle of 410° is in the first quadrant.

(b)

890° = 720° + 170°

Based on the above circular diagram, the positive angle of 890° is in the second quadrant.

(c)

$\frac{22}{9} π rad = (2 π + \frac{4}{9} π) rad = 360^{o} + 80^{o}$

Based on the above circular diagram, the positive angle of

$\frac{22}{9} π radians$ is in the first quadrant.

(d)

$\frac{10}{3} π rad = (3 π + \frac{1}{3} π) rad = 540^{o} + 60^{o}$

Based on the above circular diagram, the positive angle of

$\frac{10}{3} π radians$ is in the third quadrant.

(e)

Based on the above circular diagram, the negative angle of –60° is in the fourth quadrant.

(f)

–500° = –360° – 140°

Based on the above circular diagram, the negative angle of –500° is in the third quadrant.

(g)

$- 3 \frac{1}{4} π rad = (- 3 π - \frac{1}{4} π) rad = - 540^{o} - 45^{o}$

Based on the above circular diagram, the negative angle of

$- 3 \frac{1}{4} π radians$ is in the second quadrant.

5.2.2 Six Trigonometric Functions of Any Angle

Posted on April 22, 2020 by user

5.2b Six Trigonometric Functions of Any Angle

(B) Special Angles

(1) Value of Special Angle 30° and 60°

$\begin{array}{l} (a) \sin 30^{o} = \frac{1}{2} (b) c o s 30^{o} = \frac{\sqrt{3}}{2} (c) t a n 30^{o} = \frac{1}{\sqrt{3}} \\ (d) \sin 60^{o} = \frac{\sqrt{3}}{2} (e) c o s 60^{o} = \frac{1}{2} (f) t a n 60^{o} = \sqrt{3} \end{array}$

(2) Value of Special Angle 45°

$(a) \sin 45^{o} = \frac{1}{\sqrt{2}} (b) c o s 45^{o} = \frac{1}{\sqrt{2}} (c) t a n 45^{o} = 1$

(3) Value of Special Angle 0°, 90°, 180°, 270°, 360°

(a) y = sin x

x	0^o	90^o	180^o	270^o	360^o
sin	0	1	0	-1	0

(b) y = cos x

(c) y = tan x

x	0^o	90^o	180^o	270^o	360^o
tan	0	∞	0	∞	0

Short Question 4 – 6

Posted on April 22, 2020 by user

Question 4:
Solve the equation 3 sin A cos A – cos A = 0 for 0° ≤ A ≤ 360°.

Solution:
3 sin A cos A – cos A = 0
cos A (3 sin A – 1) = 0
cos A = 0 or sin A = ⅓

cos A = 0
A = 90°, 270°

sin A = ⅓
Basic angle = 19°28'
A = 19°28', 180° – 19°28'
A = 19°28', 160°32'

Hence A = 19°28', 90°, 160°32', 270°.

Question 5:

Solve the equation 4 sin (x – π) cos (x – π) = 1 for 0^o ≤ x ≤ 360^o.

Solution:

4 sin (x – π) cos (x – π) = 1

2 [2 sin (x – π) cos (x – π)] = 1

2 sin (x – π) cos (x – π) = ½

sin 2(x – π) = ½ ← (sin 2x= 2 sinx cosx)

sin 2(x – 180^o) = ½ ← (π rad = 180^o)

sin (2x – 360^o) = ½

sin 2x cos 360^o – cos 2x sin 360^o = ½

sin 2x (1) – cos 2x (0) = ½ ← (cos 360^o = 1, sin 360^o = 0)

sin 2x = ½

basic angle = 30^o ← (special angle, sin 30^o= ½)

2x = 30^o, 150^o, 390^o, 510^o

x = 15^o, 75^o, 195^o, 255^o

Long Question 1 & 2

Posted on April 22, 2020 by user

Question 1:

(a) Sketch the graph of y = cos 2x for 0° ≤ x ≤ 180°.

(b) Hence, by drawing a suitable straight line on the same axes, find the number of solutions satisfying the equation

$2 {sin}^{2} x = 2 - \frac{x}{180}$ for 0° ≤ x ≤ 180°.

Solution:
(a)(b)

$\begin{array}{l} 2 {sin}^{2} x = 2 - \frac{x}{180} \\ 1 - 2 {sin}^{2} x = 1 - (2 - \frac{x}{180}) \\ \cos 2 x = \frac{x}{180} - 1 \\ y = \frac{x}{180} - 1 \\ x = 0, y = - 1 \\ x = 180, y = 0 \\ Number of solutions = 2 \end{array}$

Question 2:
(a) Sketch the graph of

$y = \frac{3}{2} \cos 2 x for 0 \leq x \leq \frac{3}{2} π .$
(b) Hence, using the same axes, sketch a suitable straight line to find the number of solutions to the equation

$\frac{4}{3 π} x - \cos 2 x = \frac{3}{2} for 0 \leq x \leq \frac{3}{2} π$
State the number of solutions.

Solution:
(a)(b)

$\begin{array}{l} \frac{4}{3 π} x - \cos 2 x = \frac{3}{2} \\ \cos 2 x = \frac{4}{3 π} x - \frac{3}{2} \\ \frac{3}{2} \cos 2 x = \frac{3}{2} (\frac{4}{3 π} x - \frac{3}{2}) \\ y = \frac{2}{π} x - \frac{9}{4} \\ To sketch the graph of y = \frac{2}{π} x - \frac{9}{4} \\ x = 0, y = - \frac{9}{4} \\ x = \frac{3 π}{2}, y = \frac{3}{4} \\ Number of solutions \\ = Number of intersection points \\ = 3 \end{array}$

5.5 Formulae of sin (A ± B), cos (A ± B), tan (A ± B), sin 2A, cos 2A, tan 2A

Posted on April 22, 2020 by user

5.5 Formulae of sin (A ± B), cos (A ± B), tan (A ± B), sin 2A, cos 2A, tan 2A

(A) Compound Angles Formulae:

$\begin{array}{l} • \sin (A \pm B) = \sin A \cos B \pm \cos A \sin B \\ • \cos (A \pm B) = \cos A \cos B \mp \sin A \sin B \\ • \tan (A \pm B) = \frac{\tan A \pm \tan B}{1 \mp \tan A \tan B} \end{array}$

(B) Double Angle Formulae:

sin 2A = 2 sin A cos A
cos 2A = cos² A – sin² A
cos 2A = 2 cos² A – 1
cos 2A = 1 – 2 sin² A
$\tan 2 A = \frac{2 \tan A}{1 - \tan^{2} A}$

(C) Half Angle Formulae:

$\begin{array}{l} • \sin A = 2 \sin \frac{A}{2} \cos \frac{A}{2} \\ • \cos A = \sin^{2} \frac{A}{2} - \cos^{2} \frac{A}{2} \\ \cos A = 2 \cos^{2} \frac{A}{2} - 1 \\ \cos A = 1 - 2 \cos^{2} \frac{A}{2} \\ • \tan A = \frac{2 \tan \frac{A}{2}}{1 - \tan^{2} \frac{A}{2}} \end{array}$

5.5.1 Proving Trigonometric Identities using Addition Formula and Double Angle Formulae

Example 1:

Prove each of the following trigonometric identities.

$\begin{array}{l} (a) \frac{s i n (A + B) - s i n (A - B)}{c o s A \cos B} = 2 \tan B \\ (b) \frac{\cos (A + B)}{\sin A \cos B} = \cot A - \tan B \\ (c) \tan (A + 45^{o}) = \frac{\sin A + \cos A}{c o s A - \sin A} \end{array}$

Solution:

(a)

$\begin{array}{l} L H S \\ = \frac{s i n (A + B) - s i n (A - B)}{c o s A \cos B} \\ = \frac{(\sin A \cos B + \cos A \sin B) - (\sin A \cos B - \cos A \sin B)}{c o s A \cos B} \\ = \frac{2 \cos A \sin B}{\cos A \cos B} \\ = \frac{2 \sin B}{\cos B} \\ = 2 \tan B = R H S (proven) \end{array}$

(b)

$\begin{array}{l} L H S \\ = \frac{\cos (A + B)}{\sin A \cos B} \\ = \frac{\cos A \cos B - \sin A \sin B}{\sin A \cos B} \\ = \frac{\cos A \cos B}{\sin A \cos B} - \frac{\sin A \sin B}{\sin A \cos B} \\ = \frac{\cos A}{\sin A} - \frac{\sin B}{\cos B} \\ = \cot A - \tan B \\ = R H S (proven) \end{array}$

(c)

$\begin{array}{l} L H S \\ = \tan (A + 45^{o}) \\ = \frac{t a n A + \tan 45^{o}}{1 - t a n A \tan 45^{o}} \\ = \frac{t a n A + 1}{1 - t a n A} \leftarrow \tan 45^{o} = 1 \\ = \frac{\frac{\sin A}{\cos A} + 1}{1 - \frac{\sin A}{\cos A}} \\ = \frac{\sin A + \cos A}{\cos A} \times \frac{\cos A}{\cos A - \sin A} \\ = \frac{\sin A + \cos A}{\cos A - \sin A} \\ = R H S (proven) \end{array}$

Short Question 15 – 18

Posted on April 22, 2020 by user

Question 15:

$Prove the identity \frac{2}{\cos 2 A + 1} = s e c^{2} A$

Solution:

$\begin{array}{l} LHS \\ = \frac{2}{\cos 2 A + 1} \\ = \frac{2}{(2 \cos^{2} A - 1) + 1} \leftarrow \cos 2 A = 2 \cos^{2} A - 1 \\ = \frac{2}{2 \cos^{2} A} = \frac{1}{\cos^{2} A} \\ = s e c^{2} A \\ = RHS \\ ∴ Proven \end{array}$

Question 16:

$Prove the identity \frac{2 \tan A}{2 - s e c^{2} A} = \tan 2 A$

Solution:

$\begin{array}{l} LHS \\ = \frac{2 \tan A}{2 - s e c^{2} A} \\ = \frac{2 \tan A}{2 - (\tan^{2} A + 1)} \leftarrow \tan^{2} A + 1 = s e c^{2} A \\ = \frac{2 \tan A}{1 - \tan^{2} A} \\ = \tan 2 A \\ = RHS \\ ∴ Proven \end{array}$

Question 17:

$Prove the identity \tan x + \cot x = 2 \cos e c 2 x$

Solution:

$\begin{array}{l} LHS \\ = \tan x + \cot x \\ = \frac{\sin x}{\cos x} + \frac{\cos x}{\sin x} \\ = \frac{\sin^{2} x + \cos^{2} x}{\cos x \sin x} \\ = \frac{1}{\cos x \sin x} \leftarrow \sin^{2} x + \cos^{2} x = 1 \\ = \frac{1}{\frac{1}{2} \sin 2 x} \leftarrow \begin{array}{l} \sin 2 x = 2 \sin x \cos x \\ \frac{1}{2} \sin 2 x = \sin x \cos x \end{array} \\ = \frac{2}{\sin 2 x} \\ = 2 (\frac{1}{\sin 2 x}) \\ = 2 \cos e c 2 x \\ = RHS \\ ∴ Proven \end{array}$

Question 18:

$Prove the identity \frac{\cos x - \sin 2 x}{\cos 2 x + \sin x - 1} = \frac{1}{\tan x}$

Solution:

$\begin{array}{l} LHS \\ = \frac{\cos x - \sin 2 x}{\cos 2 x + \sin x - 1} \\ = \frac{\cos x - 2 \sin x \cos x}{(1 - 2 \sin^{2} x) + \sin x - 1} \leftarrow \cos 2 x = 1 - 2 \sin^{2} x \\ = \frac{\cos x (1 - 2 \sin x)}{\sin x - 2 \sin^{2} x} \\ = \frac{\cos x (1 - 2 \sin x)}{\sin x (1 - 2 \sin x)} \\ = \frac{\cos x}{\sin x} \\ = \cot x \\ = \frac{1}{\tan x} \\ = RHS \\ ∴ Proven \end{array}$

5.2.1 Six Trigonometric Functions of Any Angle

Posted on April 22, 2020 by user

5.2a Six Trigonometric Functions of Any Angle

(A) The definition of sin, cos, tan, cosec, sec and cot

1. Let P (x, y) be any point on the circumference of the circle with centre O and of radius r. Based on ∆ OPQ in the above diagram,

2. The definitions of tangent, cotangent, secant and cosecant of any angle are:

3. The relations of the trigonometric ratio of an angle θ with its complementary angle (90^o – θ) are:

For examples:

(a) sin 75^o= cos (90^o – 75^o) = cos 15^o

(b) tan 50^o= cot (90^o– 50^o) = cot 40^o

(c) sec 25^o= cosec (90^o – 25^o) = cosec 65^o

4. The trigonometric ratios of any negative angle (–θ) are:

A negative angle is an angle measured in a clockwise direction from the positive x-axis.
For example, – 60^ois equivalent to 300^o (360^o – 60^o).

Example:

Express each of the following trigonometric functions in terms of the trigonometric ratios of acute angles. Hence, find each value using a calculator.

(a) cos (– 325^o)

(b) tan (– 124^o)

(c) sin (– 115^o)

Solution:

(a)

cos (– 325^o)

= cos 325^o ← {The formula cos (–θ) = cos θ is used}

= cos (360^o– 325^o) ← {At fourth quadrant, cos is positive}

= cos 35^o

= 0.8192

(b)

tan (– 124^o)

= – tan 124^o ← {The formula tan (–θ) = – tan θ is used}

= – [– tan (180^o– 124^o)] ← {At second quadrant, tan is negative}

= tan 56^o

= 1.483

(c)

sin (– 115^o)

= – sin 115^o ← {The formula sin (–θ) = – sin θ is used}

= – sin (180^o– 115^o) ← {At second quadrant, sin is positive}

= – sin 65^o

= – 0.9063

5.6a Solving Trigonometric Equation (Basic Equation In Sinx/ Cosx/ Tanx/ Cosecx/ Secx/ Cotx)

Posted on April 22, 2020 by user

5.6 Simple Trigonometric Equations

Steps to solve simple trigonometric equation:
(1) Determine the range of values of the required angles.
(2) Find a basic angle by using calculator.
(3) Determine the quadrants the angle should be.
(4) Determine the values of angles in those quadrants.

(A) Solving Trigonometric Equation (Basic Equation in sinx/ cosx/ tanx/ cosecx/ secx/ cotx)

Example:
Find all the values of θ for 0° < θ < 360° that satisfy each of the following trigonometric equations.
(a) sin θ = 0.6137
(b) cos θ = 0.2377
(c) tan θ = 2.7825
(d) sin θ = -0.8537

(e) sin 2θ = 0.5293

Solution:

(a)
sin θ = 0.6137

basic angle = sinˉ¹ 0.6137 = 37.86°

θ = 37.86°, 180°-37.86°

θ = 37.86°, 142.14°

(b)
cos θ = 0.2377

basic angle = cosˉ¹ 0.2377 = 76.25°

θ = 76.25°, 360° – 76.25°

θ = 76.25°, 283.75°

(c)
tan θ = 2.7825

basic angle = tanˉ¹ 2.7825 = 70.23°

θ = 70.23°, 180° + 70.23°

θ = 70.23°, 250.23°

(d)
sin θ = -0.8537

basic angle = sinˉ¹ 0.8537 = 58.62°

θ = 180° + 58.62°, 360° – 58.62°

θ = 238.62°, 301.38°

(e)
sin 2θ = 0.5293

basic angle = 31.96°

0° < θ < 360°

0° < 2θ < 720°

2θ = 31.96°, 180° – 31.96°, 360° + 31.96°, 360° + 180° – 31.96°

2θ = 31.96°, 148.04°, 391.96°, 508.04°

θ = 15.98°, 74.02°, 195.98°, 254.02°

Short Question 9 & 10

Posted on April 22, 2020 by user

Question 9:

Given that sin θ =

$\frac{3}{5}$ , where θ is an acute angle, without using tables or a calculator, find the values of

(a) sin (180º + θ),

(b) cos (180º – θ),

(c) tan (360º + θ).

Solution:
(a)

$\sin θ = \frac{3}{5} \cos θ = \frac{4}{5} \tan θ = \frac{3}{4}$

sin (180º + θ)

= sin 180º cos θ + cos 180º sin θ

= (0) cos θ + (– 1) sin θ

= – sin θ

$- \frac{3}{5}$

(b)

cos (180º – θ)

= cos 180º cos θ + sin 180º sin θ

= (– 1) cos θ + (0) sin θ

= – cos θ

$- \frac{4}{5}$

(c)

$\begin{array}{l} \tan (360^{\circ} + θ) \\ = \frac{\tan 360^{\circ} + \tan θ}{1 - \tan 360^{\circ} \tan θ} \\ = \frac{0 + \tan θ}{1 - (0) (\tan θ)} \\ = \tan θ \\ = \frac{3}{4} \end{array}$

Question 10:

Prove each of the following trigonometric identities.

(a) cot²x – cot² x cos²x = cos² x

$(b) \frac{\sec x}{\sec x - \cos x} = \cos e c^{2} x$

Solution:

(a)

$\begin{array}{l} LHS: \cot^{2} x - \cot^{2} x \cos^{2} x \\ = \cot^{2} x (1 - \cos^{2} x) \\ = \cot^{2} x (s i n^{2} x) \\ = \frac{\cos^{2} x}{s i n^{2} x} (s i n^{2} x) \\ = \cos^{2} x (RHS) \end{array}$

(b)

$\begin{array}{l} LHS: \frac{\sec x}{\sec x - \cos x} \\ = \frac{\frac{1}{\cos x}}{\frac{1}{\cos x} - \cos x} = \frac{\frac{1}{\cos x}}{\frac{1}{\cos x} - \frac{\cos^{2} x}{\cos x}} \\ = \frac{\frac{1}{\cos x}}{\frac{1 - \cos^{2} x}{\cos x}} = \frac{1}{\cos x} \times \frac{\cos x}{1 - \cos^{2} x} \\ = \frac{1}{1 - \cos^{2} x} = \frac{1}{s i n^{2} x} \\ = \cos e c^{2} x (RHS) \end{array}$