7.1a Mean
1. The mean of the data is an average value obtained by using the formula:
Mean= Total data values Number of data
(A) Ungrouped Data
Example 1
Solution:
(a)
ˉx=2+4+7+10+13+16+187ˉx=707=10
(b)
New mean =9.570+x8=9.570+x=76x=6
1. The mean of the data is an average value obtained by using the formula:
Mean= Total data values Number of data
(A) Ungrouped Data
Example 1
(a) Find the mean for the set of data 2, 4, 7, 10, 13, 16 and 18.
(b) A value of xis added into the above set of data, the mean for this new data is 9.5. Determine the value of x.
Solution:
(a)
ˉx=2+4+7+10+13+16+187ˉx=707=10
(b)
New mean =9.570+x8=9.570+x=76x=6
(B) Grouped Data (Without Class Interval)
Example 2
Solution:
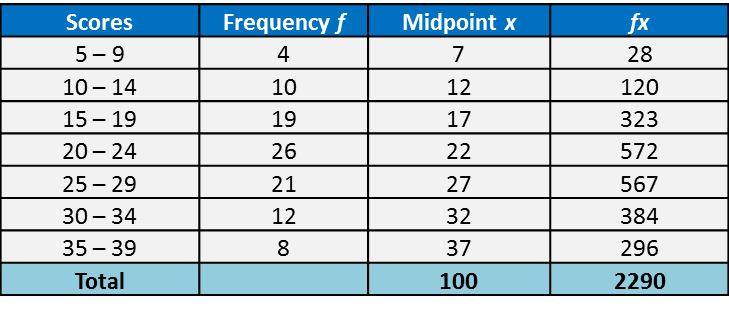
Mean marks, ˉxˉx=(50)(6)+(55)(8)+(60)(15)+(65)(10)+(70)(1)6+8+15+10+1ˉx=236040=59
Example 2
The frequency table shows the marks obtained by 40 students in a Biology test.

Find the mean marks.
Solution:
Mean marks, ˉxˉx=(50)(6)+(55)(8)+(60)(15)+(65)(10)+(70)(1)6+8+15+10+1ˉx=236040=59