Question 14 (4 marks):
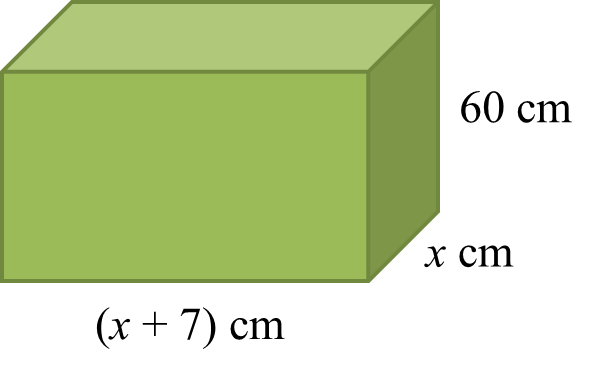
An aquarium has the length of (x + 7) cm, the width of x cm and the height of 60 cm.
The total volume of the aquarium is 48000 cm3. The aquarium will be filled fully with water.
Calculate the value of x.
Solution:
Volume of aquarium=48000 cm3So,(x)(x+7)(60) cm3=48000 cm3(x)(x+7)=4800060x2+7x=800x2+7x−800=0(x−25)(x+32)=0x−25=0 or x+32=0x=25 or x=−32(not accepted)So the value of x=25 cm
An aquarium has the length of (x + 7) cm, the width of x cm and the height of 60 cm.
The total volume of the aquarium is 48000 cm3. The aquarium will be filled fully with water.
Calculate the value of x.
Solution:

Volume of aquarium=48000 cm3So,(x)(x+7)(60) cm3=48000 cm3(x)(x+7)=4800060x2+7x=800x2+7x−800=0(x−25)(x+32)=0x−25=0 or x+32=0x=25 or x=−32(not accepted)So the value of x=25 cm
Question 15 (4 marks):
Diagram 3 shows a garden path with a rectangular shape. There are 8 similar circular stepping stone built in the path.

Given the area of the path is 32 m2, find the diameter, in m, of one piece of stepping stone.
Solution:
Given the area of the path=32 m2=320000 cm2x(x+4)=320000x2+4x−320000=0a=1, b=4, c=−320000x=−b±√b2−4ac2ax=−4±√42−4(1)(−320000)2(1)x=−4±√12800162x=−4+1131.382 or −4−1131.382x=563.69 or −567.69 (ignored)Diameter of one piece of steppingstone=x=563.69 cm=5.64 m
Diagram 3 shows a garden path with a rectangular shape. There are 8 similar circular stepping stone built in the path.

Given the area of the path is 32 m2, find the diameter, in m, of one piece of stepping stone.
Solution:
Given the area of the path=32 m2=320000 cm2x(x+4)=320000x2+4x−320000=0a=1, b=4, c=−320000x=−b±√b2−4ac2ax=−4±√42−4(1)(−320000)2(1)x=−4±√12800162x=−4+1131.382 or −4−1131.382x=563.69 or −567.69 (ignored)Diameter of one piece of steppingstone=x=563.69 cm=5.64 m
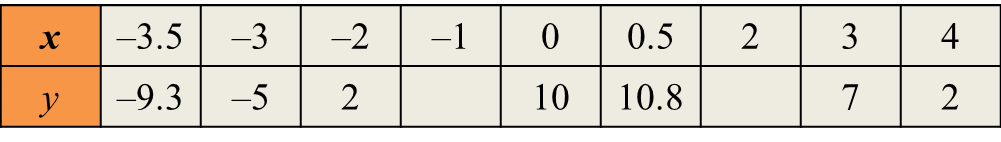
 Table 2
Table 2