Home › SPM Maths › Archive for Transformations III › Page 2

Transformations III

3.1 Combination of Two Transformations

Posted on by

3.1a Determining the image of an object under the combination of two isometric transformations
1. Translations, reflections and rotations are isometric transformations.
 
2. In an isometric transformation, the shape and the size of the image is the same as the object.
 
3. For two transformations, A and B,
(a) Combined transformation AB represents transformation B followed by transformation A.
(b) Combined transformation BA represents transformation A followed by transformation B.
(c) Combined transformation A2 = AA represents transformation A which is carried out twice consecutively.


3.1c Stating the Coordinates of the Image of a Point under a Combined Transformation

Posted on by

3.1c Stating the Coordinates of the Image of a Point under a Combined Transformation
 
1. The coordinates of the image of a point, K, under the combined transformation AB can be determined by the following steps.
 
Step 1:
Determine the coordinates of K’, the image of K, under the first transformation, B.

Step 2:
Determine the coordinates of K”, the image of K’, under the second transformation, A. K” is the image of K, under the combined transformation, AB.

Example:
T, P, R and E are four transformations that are defined as follows:
T = T r a n s l a t i o n ( − 4 3 )
P = Reflection in the y–axis.
R = Clockwise rotation 90o about the origin
E = Enlargement with scale factor of 3 and the origin as centre.
Find the coordinates of the image of the point A (3, –2) under each of the following combined transformation.
(a) TT (b) PT (c) ET (d) ER  (e) EP
 

Solution:
(a)


A(3, –2) → T → A’(–1, 1) → T → A”(–5, 4).


(b)


A(3, –2) → T → A’(–1, 1) → P → A”(1, 1).


(c)



A(3, –2) → T → A’(–1, 1) → E → A”( –3, 3).


(d)

A(3, –2) → R → A’(–2, 3) → E → A”( –6, –9).


(e)


A(3, –2) → P → A’(–3, –2) → E → A”( –9, –6).

3.1b Determining the Image of an Object under Combination of (a) Two Enlargements or (b) an Enlargement and an Isometric Transformation

Posted on by

3.1b Determining the Image of an Object under Combination of (a) Two Enlargements or (b) an Enlargement and an Isometric Transformation
 
1. Enlargement is a transformation where all points of an object on a plane move from a fixed point at a constant ratio.
 
2. The fixed point is called the centre of enlargement and constant ratio is called the scale factor.

Scale factor, k  = Length of image side Length of object side

3. For enlargement, the object and the image are similar.
 
4. Area of image = (Scale factor)2 × Area of object

= k2 × Area of object


Example:

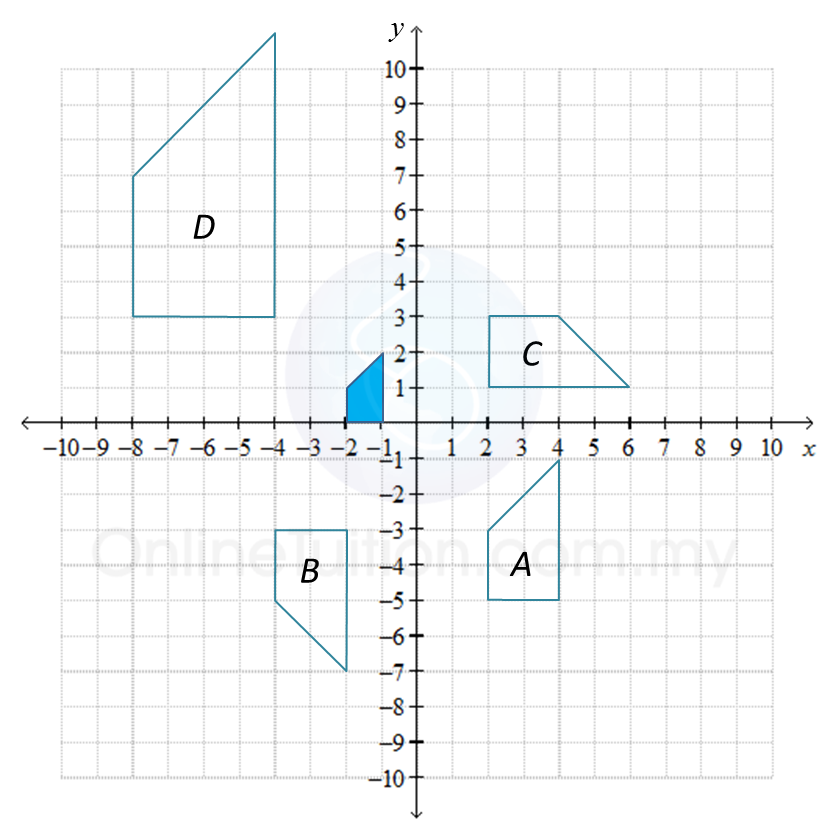
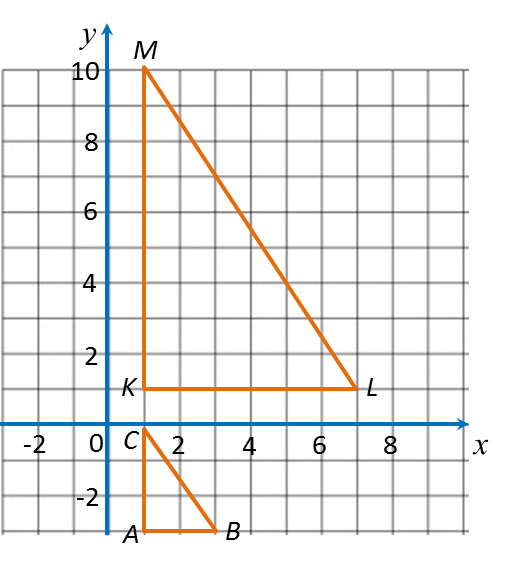
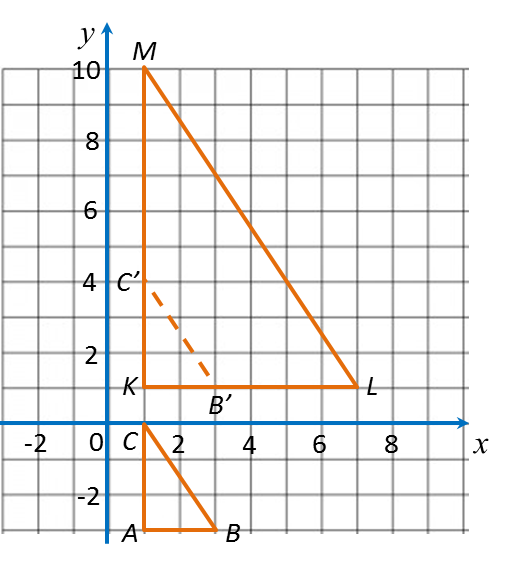
E, P and T are three transformations that are defined as follows:
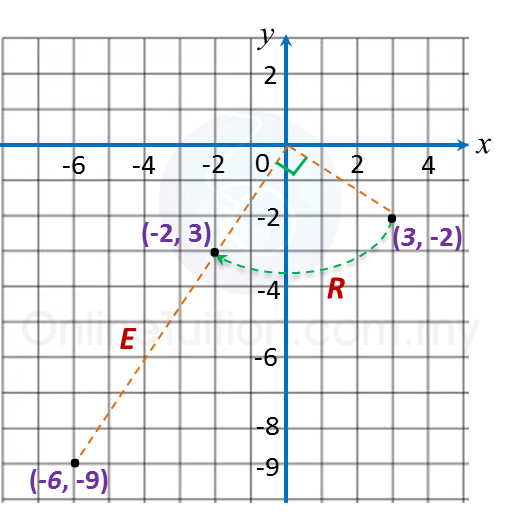
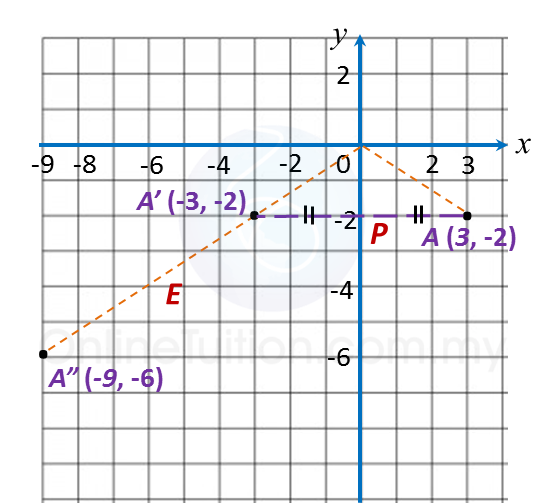
E = Enlargement with centre V (0, –1) and a scale factor of 2.
P = Reflection at the line y = –1.
T  =  T r a n s l a t i o n ( 3 − 3 )
Based on the diagram above, determine the image of the shaded figure under the combined transformations
(a) E2  (b) ET (c) EP
 

Solution:

(a)
Shaded figure → (E) figure III → (E) figure D.
Hence, the image of the shaded figure under the combined transformation E2 = EE is the figure D.
 
(b)
Shaded figure → (T) figure II → (E) figure A.
Hence, the image of the shaded figure under the combined transformation ET is the figure A.
 
(c)
Shaded figure → (P) figure I → (E) figure B.
Hence, the image of the shaded figure under the combined transformation EP is the figure B.

3.1d Describing the Combination of Two Transformations Given the Object and the Image

Posted on by

3.1d Describing the Combination of Two Transformations Given the Object and the Image
 
Example 1:

In the diagram above, triangle KLM is the image of triangle ABC under the combined transformations VW. Describe in full, the transformation W and the transformation V.

Solution:


Figure ABC → W→ Figure KB’C’ → V → Figure KLM

W = Translation ( 0 4 )   
V= Enlargement with centre K(1, 1) and a scale factor of 3.



Example 2:


In the diagram above, triangle CKL is the image of triangle ABC under the combined transformations UY. Describe in full, the transformation Y and the transformation U.
 
Solution:


Figure ABC → Y→ Figure AB’C → U → Figure CKL
Y = Reflection in the straight line y = 6
U = Enlargement with centre C(12, 6) and a scale factor of 2.



Example 3:


In the diagram above, triangle PQR is the image of triangle ABC under the combined transformations TW. Describe in full, the transformation W and the transformation T.
 
Solution:



Figure ABC → W→ Figure PBC’ → T → Figure PQR
W = Clockwise rotation of 90o about the point B (5, 5)
T = Enlargement with centre P (1, 5) and a scale factor of 2.

3.2 Solving Problems involving Transformations

Posted on by

3.2 Solving Problems involving Transformations

Example:
The diagram below shows the triangles ABC, SQT and PQR.



(a) Transformation T is a translation (  5 −2 )  
Transformation P is a reflection in the straight line y = 7.
State the coordinates of the image of point A under each of the following transformations:
(i) Transformation T,
(ii) Combined transformation PT.

(b)
(i) PQR is the image of triangle ABC under the combined transformations MN. Describe in full, the transformation N and the transformation M.
(ii) Given that the area of the shaded region PSTR is 200 m2, calculate the area of the triangle SQT.

Solution:
(a)


(i) A(3, 11) → T → A’(8, 9).
(ii) A(3, 11) → T → A’(8, 9) → P → A”(8, 5).


(b)(i)

N = Anticlockwise rotation of 90o about the point B(5, 10).


(b)(ii)


M = Enlargement with centre (5, 12) and a scale factor of 3.


(c)
Area of image = (Scale factor)2 x Area of object
Area of PQR = 32 x Area of SQT
Area of SQT + Area of PSTR = 9 x Area of SQT
Area of SQT + 200 = 9 x Area of SQT
200 = 9 x Area of SQT – Area of SQT
200 = 8 x Area of SQT
Area of SQT = 200 8
Area of SQT = 25 m2